1
$\begingroup$

I have a system $$f_1(x_1,\dots,x_m) = 0,...,f_p(x_1,\dots,x_m) = 0,$$ where $f_1,\dots,f_p$ are real polynomials and $x_1,\dots,x_m$ are real independent variables. I'm interested in an upper bound for the number of connected components of the zero set of my system.

I have the following argument using the Thom-Milnor but I'm unsure if it's correct. (The main problem is that I'm completely incompetent at algebraic topology.) Here is the theorem I want to use.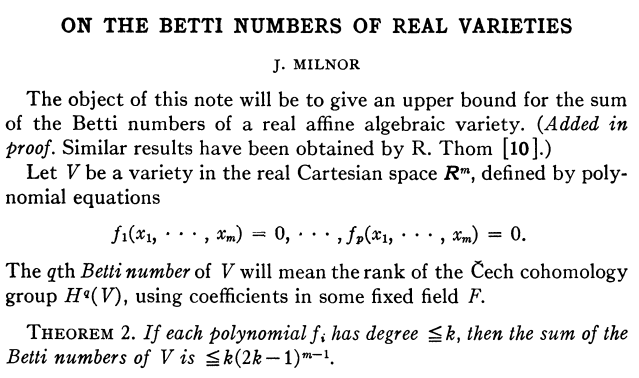

So I have that the sum of Betti numbers is $\le k(2k-1)^{m-1}$. Now every Betti number is non-negative since it's a rank of some group and the $0$th Betti number $b_0$ is the number of the connected components. So we can conclude that the number of connected components of the zero set is $\le k(2k-1)^{m-1}$.

Is this a correct argument? In particular:

  1. there is a word variety in the formulation of the theorem. Does it imply that $f_1,\dots,f_p$ should be good in some way? There is this irreducibility condition in some definitions which would be a deal breaker I guess. Or this works for any $f_1,\dots,f_p$?
  2. Wikipedia says that $b_0$ is the number of connected components in simplicial complexes. Is this still true for our zero set?
Improve this question
$\endgroup$
1
  • 2
    $\begingroup$ Yes, your argument is correct, I am not sure about your question 1, but a quick look at the proof seems to indicate that it should work for any polynomials. As to 2, yes, it is true except for "very bad" sets (from view point of classical algebraic topology), and affine varieties are always good enough. $\endgroup$
    – user43326
    Commented Mar 16, 2023 at 8:52

0

Reset to default

You must log in to answer this question.