Let $(X_t, t \ge 0)$ be a $\mathbb R^d$-valued stochastic process. Let $\lambda>0$. Assume we have $\mathbb E [|X_0|^2] < \infty$ and $$ \mathbb E [|X_t|^2] - \mathbb E [|X_0|^2] \le -2 \lambda \int_0^t \mathbb E [|X_s|^2] \, \mathrm d s + 2t (\lambda |\mathbb E [X_0]|^2 +1) \quad \forall t \ge 0. $$
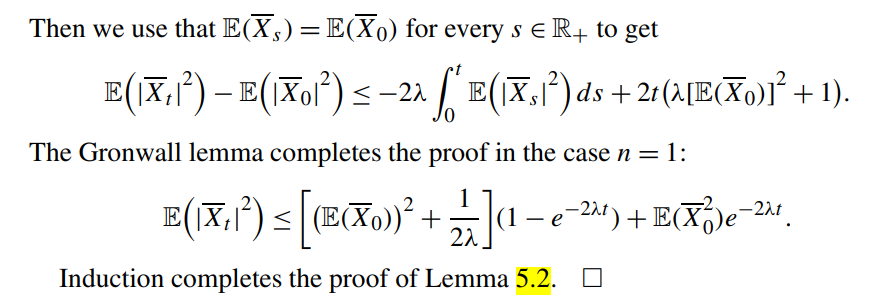
It is mentioned in the proof of Lemma 5.2 of the paper Convergence to equilibrium for granular media equations and their Euler schemes that
By Gronwall's lemma, $$ \mathbb E [|X_t|^2] \le \bigg [ |\mathbb E [X_0]|^2 + \frac{1}{2\lambda} \bigg ] (1- e^{-2\lambda t}) + \mathbb E [|X_0|^2] e^{-2\lambda t}. $$
Could you explain which form of the Gronwall lemma is used in the paper?
On the other hand, the form of Gronwall lemma on Wikipedia seems not applicable in this case, i.e.,
Let $I$ denote an interval of the real line of the form $[a, \infty)$ or $[a, b]$ or $[a, b)$ with $a<b$. Let $\alpha, \beta$ and $u$ be realvalued functions defined on $I$. Assume that $\beta$ and $u$ are continuous and that the negative part of $\alpha$ is integrable on every closed and bounded subinterval of $I$.
- (a) If $\beta$ is non-negative and if $u$ satisfies the integral inequality $$ u(t) \leq \alpha(t)+\int_a^t \beta(s) u(s) \mathrm{d} s, \quad \forall t \in I, $$ then $$ u(t) \leq \alpha(t)+\int_a^t \alpha(s) \beta(s) \exp \left(\int_s^t \beta(r) \mathrm{d} r\right) \mathrm{d} s, \quad t \in I. $$
- (b) If, in addition, the function $\alpha$ is non-decreasing, then $$ u(t) \leq \alpha(t) \exp \left(\int_a^t \beta(s) \mathrm{d} s\right), \quad t \in I. $$
Below is the screenshot I took from the paper.