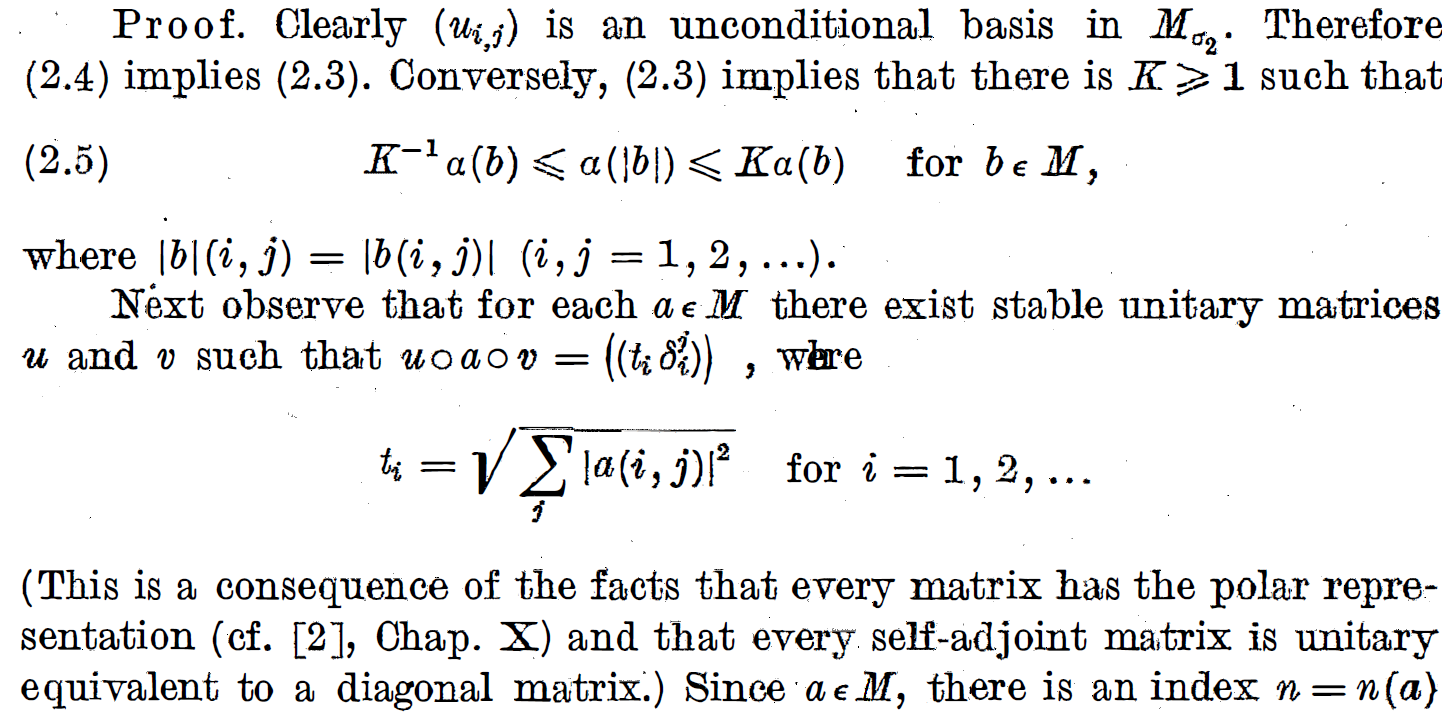1
$\begingroup$

I am reading an old paper by Kawpien and Pelczynski, Studia Math. 1970. It claims that singular values of a matrix (with positive entries? I am not sure) is given by $t_i=\sqrt{\sum_{j\ge 1}a(i,j)^2}$. I am not sure why we have such a nice characterization of singular values of a matrix...

https://eudml.org/doc/217431

enter image description here

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
2
$\begingroup$

This is clearly a mistake, for example the singular values of the matrix $\begin{pmatrix} 1 & 1\\1&1\end{pmatrix}$ are $(2,0)$ and not $(\sqrt 2,\sqrt 2)$.

But it is harmless here, as the only thing that is used later in the proof is that $\sum_i t_i^2 = \sum_{i,j} |a_{i,j}|^2$, which is obvious.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I see...I thought I have missed something here...Thank you very much! $\endgroup$
    – user92646
    Commented Dec 2, 2022 at 9:21

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .