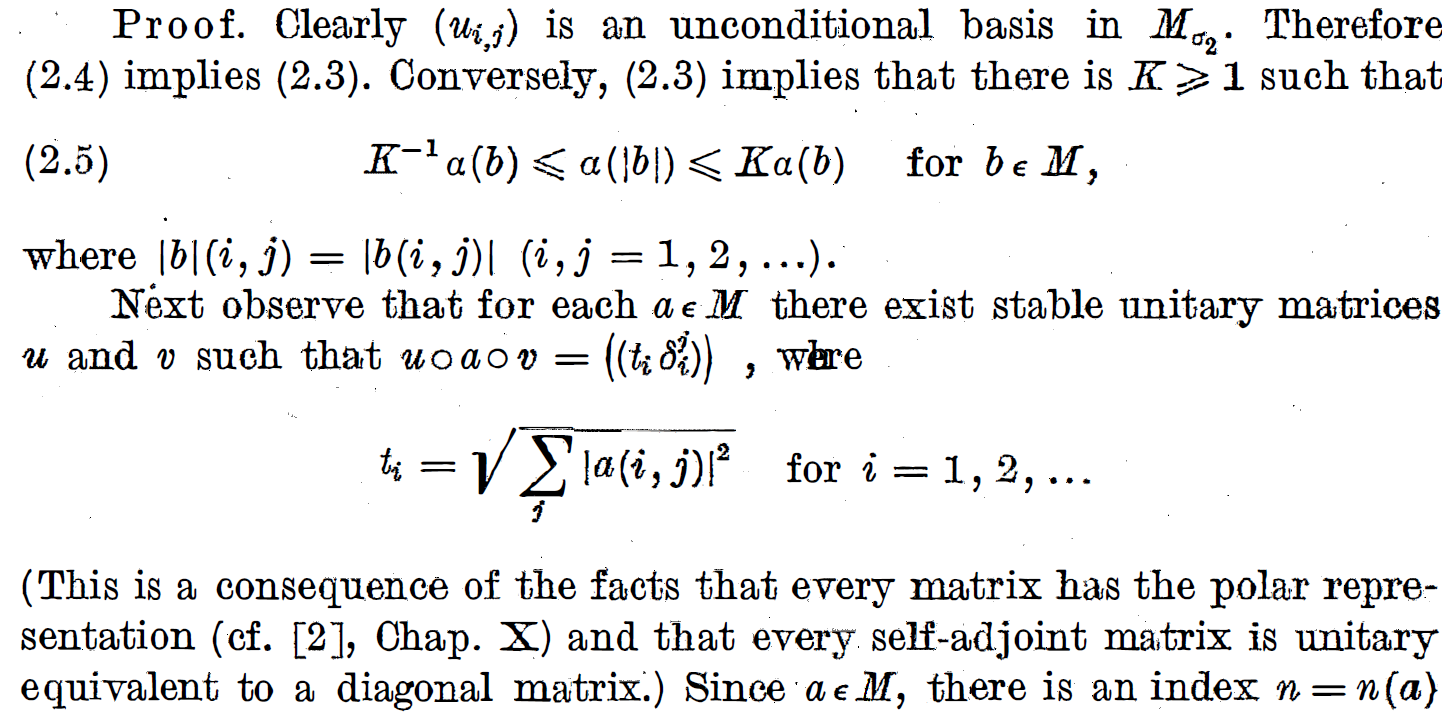I am reading an old paper by Kawpien and Pelczynski, Studia Math. 1970. It claims that singular values of a matrix (with positive entries? I am not sure) is givngiven by $t_i=\sqrt{\sum_{j\ge 1}a(i,j)^2}$. I am not sure why we have such a nice characterization of singular values of a matrix...