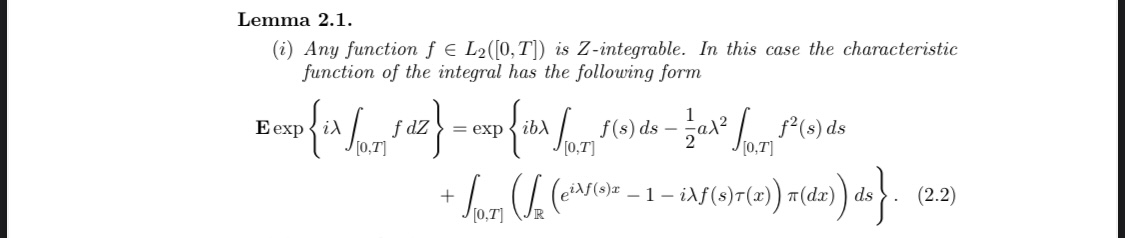0
$\begingroup$

I have a question about the second moment of the integral wrt Levy Processes.

Let Z a Levy processe. We know that:

enter image description here

And a few page later is written that by differentiation of the characteristic function above (2.2) we can deduce that the integral admist second moment, in fact we have : enter image description here

Can anyone explain to me how to calculate it, or can they at least give me a hint? I have to differentiate, and then?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

For any random variable $Y$ with a finite second moment, one has $$ \left.\frac{\partial^2}{\partial \lambda^2}\mathbb{E} e^{i\lambda Y}\right|_{\lambda=0} =\left. \mathbb{E}\frac{\partial^2}{\partial \lambda^2} e^{i\lambda Y}\right|_{\lambda=0}=-\mathbb{E} Y^2. $$ The exchange of derivative and the expectation can be justified by dominated convergence theorem. The converse is also true: if the characteristic function is twice differentiable at the origin, then the second moment exists. See e.g. Durrett, Probability: theory and examples, Theorem 3.3.21.

The explicit expression for the second moment is obtained by expanding the RHS of (2.2) in a Taylor series in $\lambda$ and picking out the coefficient at $\lambda^2$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I forgot the formula you wrote to me, thank you very much! I tried to do the steps and, if it weren't for some "2" more that come out developing with taylor, the formula comes back to me. I'll try to double-check the steps more calmly. Thank you! $\endgroup$
    – Ginger 17
    Commented Nov 29, 2022 at 11:01

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .