$\zeta(2n)$ and Levy processes

Question

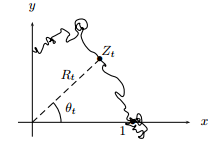
I am missing some steps in the final derivation of a probabilistic computation of the even values of $\zeta$. They show the Cauchy distribution is relate to a certian Levy process: $$ |\mathbb{C}_1| \stackrel{\text{law}}{=} e^{\frac{\pi}{2} \hat{C}_1} \text{ with }\mathbb{E}\left[ e^{i\lambda \hat{C}_1} \right] = \frac{1}{\cosh \lambda} $$ If $X_t$ and $Y_t$ be $\mathbb{R}$-valued Brownian motions, then we have a $\mathbb{C}$-valued Brownian motion. $$ Z_t = X_t + i Y_t \text{ with }Z_0 = 1+0i$$ If we switch to polar coordinates, there is a "time-change" such that it is still Brownian motion, e.g. by conformal invariance of Brownian motion, as discussed in these early chapters in SLE.

• $\log R_t = \beta_{H_t} $ ($\log R_t$ is not a Brownian motion)
• $\theta_t = \gamma_{H_t} $

Then thy considered the hitting time for the Y-axis: $T = \text{inf} \{ t: X_t = 0 \}$. In the time-change parameterization: $$ H_T := T^{\gamma, *}_{\pi/2} $$ The $Y$-corrdinate at the hitting time $T$ (or $H_T$) is distributed as the Cauchy distribution.

\begin{eqnarray} \log | \mathbb{C}_1 | &\stackrel{\text{law}}{=}& \beta_{T^{\gamma, *}_{\pi/2}} \\ \frac{2}{\pi} \, \log | \mathbb{C}_1 | & \stackrel{\text{law}}{=} & \beta_{T^{\gamma, *}_{1}} \end{eqnarray}

The log-radius and angle $\beta$ and $\gamma$ are independent Brownian motions, over time. I was not able to varify this change of equations:

$$ \mathbb{E} \left[ e^{i\lambda \frac{2}{\pi} \log | \mathbb{C}_1|} \right] \stackrel{1}{=} \mathbb{E} \left[ e^{i\lambda \beta_{T^{\gamma,*}_{\pi/2}} } \right] \stackrel{2}{=} \mathbb{E} \left[ e^{ - \frac{\lambda^2}{2} T_1^{\gamma, *} } \right] \stackrel{3}{=} \frac{1}{\cosh \lambda} $$

I wish the authors would come up with different variable names for the hitting times.

Why 2 and 3 are correct? And can anyone explain the bigger picture of why these authors feel special values of zeta might be connected to these random processes?

---

Reference:

• Euler's formulae for $\zeta(2n)$ and products of Cauchy variables Paul Bourgade, Takahiko Fujita, Marc Yor

Answer 1

The (2) is just the independence of the Brownian motion $ (\beta_t)_t $ with the ``clock'' $ (H_T)_T $. This is a classical result due to Lévy (and more generally, this is the Dambis-Dubins-Schwartz representation theorem that any continuous martingale is a changed-time Brownian motion, the BM being independent of the time change). You can find it the book by Revuz and Yor. You use the scaling of the BM and the fact that $ \beta_1 $ is a Gaussian to get this $ \frac{\lambda^2}{2} $.

The (3) comes from the first equality you gave. You should better write

$$ \frac{1}{\cosh \lambda} \stackrel{0}{=} \mathbb{E} \left[ e^{i\lambda \frac{2}{\pi} \log | \mathbb{C}_1|} \right] \stackrel{1}{=} \mathbb{E} \left[ e^{i\lambda \beta_{T^{\gamma,*}_{\pi/2}} } \right] \stackrel{2}{=} \mathbb{E} \left[ e^{ - \frac{\lambda^2}{2} T_1^{\gamma, *} } \right] $$

and now, it is clear that (0) is the first equality.

I had a look at the article : the link with the special values of $ \zeta $ comes from an explicit expression for the density of $ \mathbb{C}_1 \mathbb{C}_2 $. The goal of the article is to provide a probabilistic proof of the identities (1) and (2) (in the article) using the planar BM. It appears that this is related with some stopping of the BM on a line after the computations given in the article. For the intuition, though, I can't really say anything.