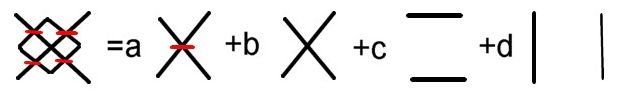You can interpret a featureless graph as product of featureless abstract tensors; the tensors are then automatically totally symmetric as "leg crossing" in the graph interpretation is the identity.
Now "featureless graph" is by no means forced, you e.g. can color vertices (multiplicities!), color edges (irreps!) and the vertices don't have to be featureless (see over- and undercrossings of a knot): Assume all your (tetravalent) vertices induce antisymmetric tensors $T$. This will be fully compatible with (knot polynomials of) the $E_7$ series of Lie algebras, since, as you can easily count that, with $V$ being the defining irrep, $dim\ V^{\otimes4}=4$ (four independent tensors: $T$ and, using ASCII art, ||,X,=; you now have to postulate that a 2-loop of two $T$ tensors can be reduced). And you can construct exactly one $T$ from a trivalent node (again ASCII art >= , single line $V$, double line adjoint $A$), again with some natural symmetry assumptions - it's just a quartic Casimir.
With no further assumptions, as it is easy to count again, $dim\ V^{\otimes6}>=40$ (> if further irreducible 6-tensors exist). With some decent additional assumption you can now force the result into one of the members of the $E_7$ series. For example any version of the Jacobi relation works (this amounts to killing a 5-dimensional irrep of the permutation group $S_6$), since then also all 3-loops are reducible too.
But what if you don't assume anything further than $dim\ V^{\otimes4}=4$? Has anybody tried to check if this forces the Jacobi relation "through the backdoor", by just piling up antisymmetric 4-tensors $T$ and reducing the graph in different ways? Simplest example (no 2-loops, but reducible by assumption, $T$ symbolized by red bar), but this probably gives no new equations:
Endnote: Since 40 years I now try to prove or disprove a statement amounting to "there is a selfconsistent $E_7$ plane just as the Vogel $E_8$ plane, also implying the objects behind it are some generalization of a Lie algebra, and there is a 3-variable knot polynomial associated with it". The profis are finally coming close too, as it seems: cubic Hecke