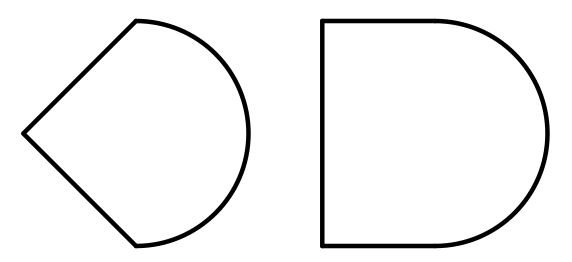2
$\begingroup$

Call a convex body $C\subset\Bbb R^n$ semi-algebraic if it can be written as

$$(*)\quad C=\bigcap_{i\in I}\, \{x\in \Bbb R^n\mid p_i(x)\le 0\}$$

with polynomials $p_i\in\Bbb R[X_1,...,X_n]$ and a finite index set $I$.

Question: Is the polar dual $C^\circ$ of a semi-algebraic convex body again semi-algebraic?

Where polar dual means

$$C^\circ := \{y\in\Bbb R^n\mid \langle x,y\rangle\le 1\text{ for all $x\in\Bbb R^n$}\}.$$

Improve this question
$\endgroup$
2
  • 4
    $\begingroup$ Have you tried using Tarski-Seidenberg, in particular in its quantifier-elimination form? $\endgroup$
    – Robert Furber
    Commented Aug 1, 2022 at 11:29
  • $\begingroup$ @RobertFurber Thank you very much! This is new territory for me, so I wasn't aware of this theorem. But I was able to use it, see my answer. There is just some detail I can't extract from the theorem: why is $C^\circ$ of the form $(*)$ rather than some semi-algebraic set that requires set-unions? I can imagine that this is where I need to use convexity. $\endgroup$
    – M. Winter
    Commented Aug 1, 2022 at 12:28

1 Answer 1

Reset to default
2
$\begingroup$

The comment of Robert brought me onto the right track. Say $I=\{1,...,m\}$, then

\begin{align} \Bbb R^n\setminus C^\circ &= \{\,y\in\Bbb R^n\mid \exists x\in C\colon\langle x,y\rangle >1\,\} \\&= \{\,y\in\Bbb R^n\mid \exists x\in\Bbb R^n\colon p_1(x)\le 0 \land\cdots\land p_m(x)\le0 \land \langle x,y\rangle>1\,\} \\&=\pi\, \{(x,y)\in\Bbb R^n\times\Bbb R^n\mid p_1(x)\le 0 \land\cdots\land p_m(x)\le0 \land \langle x,y\rangle>1\,\}, \end{align}

where $\pi$ is the projection $(x,y)\mapsto y$.

So $\Bbb R^n\setminus C^\circ$ is the projection of a semi-algebraic set. By Tarski-Seidenberg $\Bbb R^n\setminus C^\circ$ is a semi-algebraic set, and so is its complement $C^\circ$.

What I don't yet see is why it is necessarily of the form $(*)$, i.e. using only intersections. In fact, I think the following pair of shapes (which are polar duals of each other) forms a counterexample:

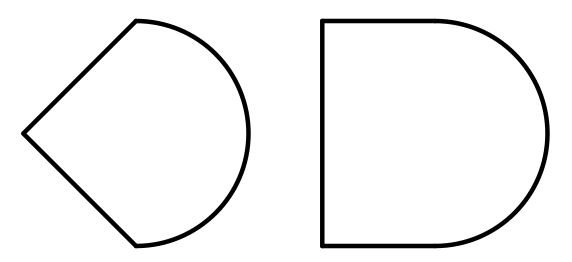
The shape on the left is semi-algebraic convex in my sense, being the intersection of a disc and two halfspaces. However, I can't see how the shape on the right can be written as an intersection of algebraic sets.

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ Indeed, the shape on the right is not an intersection of polynomial inequalities. One of the inequalities would have to vanish on an infinite subset of the circular arc, and to odd order, at which point it also vanishes to odd order on the part of the circle in the interior of your shape. (This is probably why your definition of semialgebraic is not the standard one. :)) $\endgroup$
    – David E Speyer
    Commented Aug 1, 2022 at 13:19
  • 1
    $\begingroup$ I recently read ams.org/journals/proc/2012-140-04/S0002-9939-2011-10989-4/… , so I now know that, what M. Winter is calling "semialgebraic" is usually called "basic semialgebraic". $\endgroup$
    – David E Speyer
    Commented Aug 5, 2022 at 17:51

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .