Primes and chirality: a definition and question in the context of tessellations for squares

Question

These days I'm trying to research relations between prime numbers and the notion of chirality in the $xy$-plane. Wikipedia has the article Chirality.

I don't know if this relation or the problem for which I ask here is in the literature; please add a comment in such case.

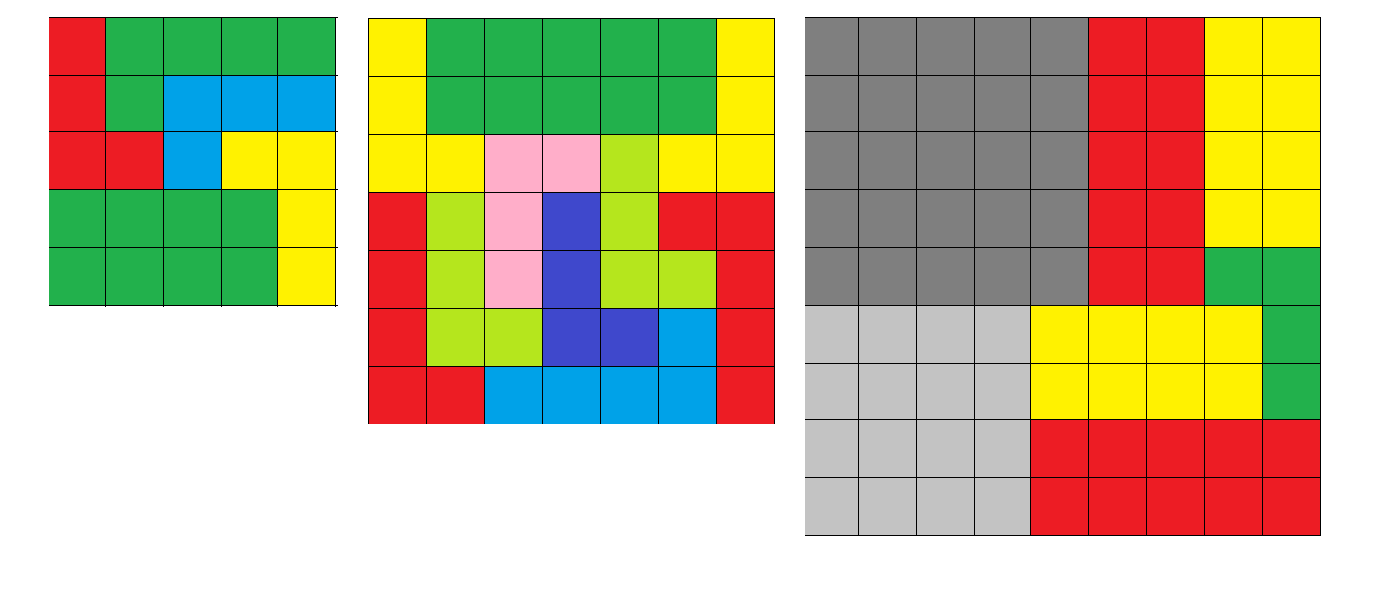
Definition. In this post, I consider a definition ad hoc, defining as admissible polygons $P$ the connected regions in the $xy$-lattice that are arrangements of unit squares with $\text{area}(P)=p>3$ a prime number, and also by definition I consider that these polygons must have a chiral shape, that is these pieces are chirals. A tessellation of a connected region $R$ by means of admissible polygons is called an admissible tessellation of $R$ (in our problem $R=S$ will be a square $S$ in the $xy$-lattice).

(The rationale for previous definitions is that any polygonal representation of the integers $1,2$ or $3$ aren't chirals, and on the other hand, again by definition one can consider that a given composite integer can be represented as a rectangle or square $A\times B$, that isn't chiral polygon, of sides $1<A\leq B$ in the $xy$-lattice as an arrangement of $n=A\cdot B$ unit squares. I emphasize with these brackets that these pieces will not be used in the tessellations in this post).

Conjecture. There exists a constant $N_0$ such that every square $S=N\times N$ of side $N$ with $N>N_0$ can be represented by admissible polygons.

Question. I would like to know what work can be done to prove the veracity of the previous conjecture. Many thanks.

With admissible polygons it is easy get admissible tessellations for the square $4\times 4$, $5\times 5$, $6\times 6$ and $7\times 7$. We have the following obvious statement.

Proposition. Given an admissible tessellation for the square $S=N\times N$ of $N^2$ unit squares, it is immediate to get an admissible tessellation for the square $\hat{S}$ of side $2N$, since this second square $\hat{S}$ admits the obvious decomposition into four squares $S$.

Thus we must deal with squares $S=N\times N$ with $N>7$ an odd integer. Exploiting a specialization of a well-known identity $1+3+5=3^2$ I can tessellate the square $9\times 9$, because this square can be split as two copies of the square $(1+3)\times(1+3)$, plus the tessellated region corresponding to the square $5\times 5$ plus a region that can be tessellated with two admissible polygons with areas $7$ and $17$, being these chirals (I just emphasize it to provide details).

I think that the conjecture isn't obvious.

If you have problems getting it, and it is required, I can add in a figure some of the mentioned tessellations.

Answer 1

I claim that there is an $N$ so that any rectangle with both sides at least $N$ can be decomposed into squares of sides $4,5,6$ and $7.$ If we show that, then the same applies squares of sides at least $N.$ I will show this for $N=1178.$ With a little more work that number could be decreased to $90.$ Although that is probably not optimal.

Let $a,b>0$ be relatively prime integers.

It is a result of Frobenious that any integer $m\geq f(a,b)=ab-a-b+1$ can be written in the form $m=as+bt$ with $s,t$ non-negative.

Using just $a\times a$ squares we can make a rectangle $a\times ab$ and using just $b \times b$ squares we can make a rectangle $b \times ab.$ Using those blocks we can make a rectangle $m \times ab$ for any $m\geq f(a,b)=ab-a-b+1$

Hence

• Using $4 \times 4$ and $5 \times 5$ squares we can make a rectangle $20 \times m$ for $m \geq f(4,5)=12.$

-Using $7 \times 7$ and $9 \times 9$ squares we can make a rectangle $63 \times m$ for $m \geq f(7,9)=48$

• Using all four sizes of squares we can make any rectangle $m \times n$ provided that $m \geq 48$ and $n \geq f(20,63)=1178.$

Sticking to squares and rectangles which can be decomposed into vertical rows each of which itself can be decomposed into horizontal columns of widths $4,5,6,7$ or $9$ (with the further restriction that each row uses only two widths) , is probably far from optimal. However, by the method above we can get

• Any row $2x \times 2y$ with $\min(2x,2y) \geq 12$ using $(a,b)=(4,6).$
• Any row $3x \times 3y$ with $\min(3x,3y) \geq 18$ using $(a,b)=(6,9).$
• Any row $ab \times m$ with $m \geq f(a,b)=ab-a-b+1$ for any of the eight relatively prime pairs drawn from $\{4,5,6,7,9\}$

Ignoring the first two types, we can get any rectangle $p \times q$ with with $p \in \{20,28,30,35,36,42,45,63\}$ and $q \geq 48.$

Combining those we can get any rectangle $r \times q$ with

$r \in \tiny{\{ 20, 28, 30, 35, 36, 40, 42, 45, 48, 50, 55, 56, 58, 60, 62, 63, 64, 65, 66, 68, 70, 71, 72, 73, 75, 76, 77, 78, 80, 81, 82, 83, 84, 85, 86, 87, 88\}}$ or $r \geq 90$ and $q \geq 48.$

Answer 2

Here is a different answer. You mention a particular interest in the $L$-$n$omino: a column of $n-1$ squares with one more square to the right on the bottom row. It has $4$-rotations and another $4$ obtained by rotation and reflection. The reflection might be called the $J$-$n$omino if one wanted to distinguish rotations from reflections (more often, people do not.) It is true that $n-1=\varphi(n)$ for $n$-prime, but I don't see that you have motivated your focus on primality.

I will conjecture that if $\gcd(m,n)=1$ then using just the $L$-$m$omino and $L$-$n$omino you can tile any rectangle with both sides above some $N=N(m,n).$ In fact it might be that you could make do with just one orientation of each one in all but a few cases (that is to say, $L$s but not $J$s). But then you would need a larger $N.$

In spite of your disinterest in tiling with rectangles, that is the key. Once you have a few rectangles you can use those as blocks to make others.

A coloring argument shows that any rectangle tiled by the $L$-tetromino uses an even number so has area a multiple of $8$. One easily finds how to get a $2\times 4$ and once you know to look for a $3\times 8$ you can find that too.

Aside: These two are called the primes of this tetromino because any other rectangle you can get can be made from them.

Claim: any rectangle with area a multiple of $8$ and shortest side at least $2$ can be tiled.

Sketch: if both sides are even, the $2 \times4$ alone is sufficient. If one side is odd, the other is a multiple of $8$. Stacking $k-1$ $2 \times 8$ and a single $3 \times 8$, you can get $(2k+1) \times 8$

You can find a great deal of information about rectangle tilings at this page

For the $L$-pentomino one finds figures showing that the $2 \times 5$ and $7 \times 15$ can be tiled

Claim: Any rectangle with area a multiple of $5$ can be tiled as long as the one side is at least $15$ and the other at least $7.$

Using just the $2 \times 4$ and $2 \times 5$ you can get any $2 \times k$ except for $k=1,3,6,7,11$ and from that any even area rectangle with shortest side at least $12.$ One could probably improve that.

And using just those two $L$-ominos and the four prime rectangles mentioned, one can get a $15 \times k$ and an $8 \times k$ for any $k \geq 7$ and hence also a $j \times k$ as long as $j \geq 98.$ Probably much better results are possible with a little work.

A link on that pentomino page leads to the claim (perhaps with illustrations proving it) that using only the $L$ (and not the $J$) one can get $2\times 5, 13\times 55, 15\times 39, 17 \times 35$ and $19 \times 25.$ Those are the one handed primes and with them you can get any rectangle with area a multiple of $5$ as long as the shortest side is at least $19$.

A LITTLE MORE WORK

We know how to get all squares which are of even side at least $4$ using just the $L$-teromino (no $J$-tetrominos)

Here are a $5 \times 5, 7 \times 7$ and $9 \times 9$. The solid rectangles are all ones which we know we can fill. For what it is worth, the $5 \times 5$ uses only $L$'s. I did not try to do that for the rest, it might not be hard. In fact the $9 \times 9$ has that property,

With four $3 \times 8$ rectangles around a $5 \times 5$ square we can make an $11 \times 11$ square.

Finally, if $k \geq 5$ is odd then we can make a a square of side $k+8$ by using a $k\times k$ in the upper left, and $8 \times 8$ on the lower right, a $k \times 8$ in the upper right and an $8 \times k$ in the lower left.