Reference request for a certain exponential series

Question

I recently encountered the series $$\sum_{d \in \mathbb{Z}} e^{-t^d}t^{kd},$$ for real $0<t<1$ and $k$ a positive integer. It converges because roughly speaking, when $d$ is large and positive the terms are about $t^{kd}$, and when $d$ is large and negative $e^{-t^d}$ is very small. Has it or a series like it been studied in the literature to anyone’s knowledge, and are there reasonable alternative representations of it?

Answer 1

Q: Is there a reasonable alternative expression for the sum $$\sum_{d=-\infty}^\infty e^{-t^d}t^{kd},\;\;0<t<1,\;\; k\in\mathbb{N}.$$ A: I note the integral expression $$\int_{-\infty}^\infty e^{-t^x}t^{kx}\,dx=-\frac{(k-1)!}{\ln t}.$$ This is a reasonable approximation to the sum when $t$ is not much smaller than 1 and $k$ is a small integer. For $k=1$, in particular, sum and integral are nearly indistinguishable for $t\gtrsim 0.2$. If $t\gtrsim 0.5$ one can go up to $k=8$.

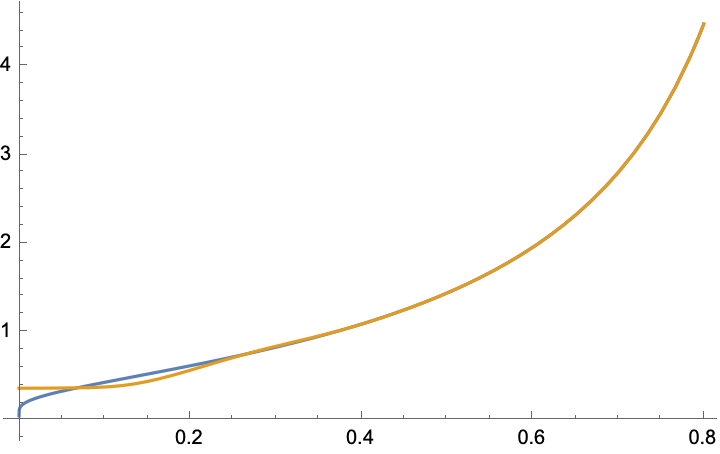
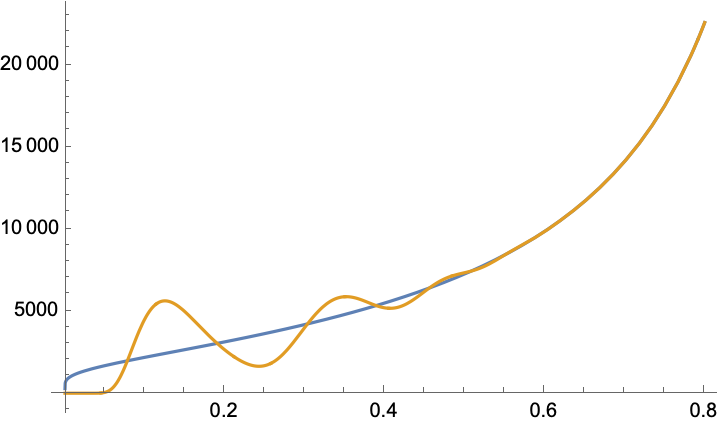
Sum (gold) versus integral (blue) as a function of $t$, for $k=1,2,4,8$. The oscillations should follow from the first few terms of the Poisson summation (the integral being the zeroth term). I have not succeeded in evaluating these.