I was recently looking at the figure eight knot complement $M$, as a once-punctured torus bundle over the surface with monodromy $ A=\begin{pmatrix} 2 & 1 \\ 1 & 1 \end{pmatrix} $ and its sister with monodromy $-A$.
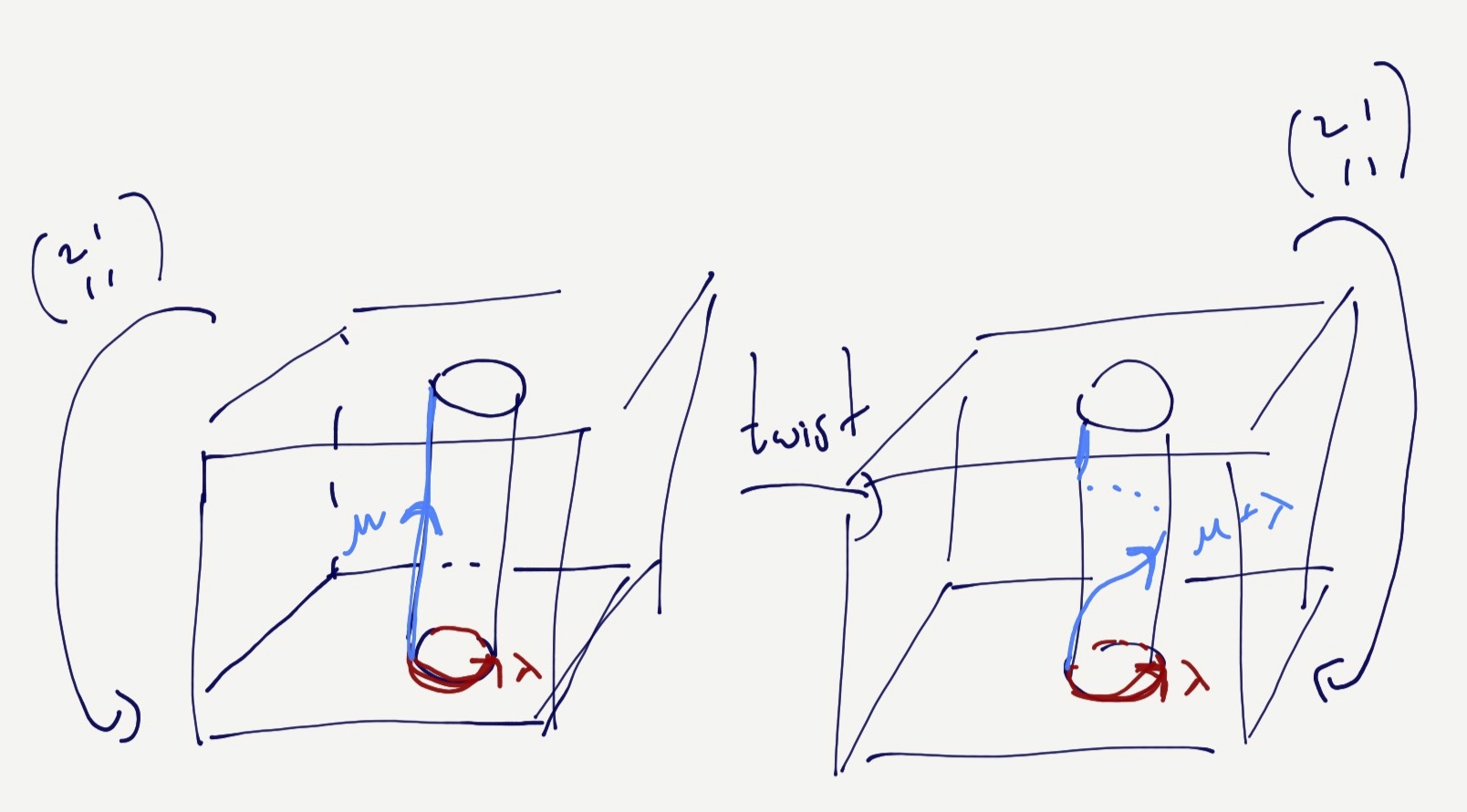
I'm confused about the following: Suppose I take this bundle, with peripheral subgroup: $\lambda$ the boundary of the torus, and $\mu$ the meridian, which is vertical in my bundle picture. Now suppose I twist the top of the bundle in my picture by a full twist of $2\pi$ before gluing. I can't seem to reconcile the following three "facts":
- Since I didn't actually change the monodromy I clearly get a self-homeomorphism of $M$.
- This homeomorphism takes $\lambda$ to itself and $\mu$ to $\mu+\lambda$.
- Such a homeomorphism doesn't exist as any homeomorphism will have to take $\mu$ to $\pm\mu$ by a theorem from ``knots are determined by their complements" by Gordon–Luecke.
So then, which one is wrong? Any help appreciated!