Let $S$ be closed hyperbolic surface with genus $g\geq 2$. Let $Teich(S)$ be the Teichmueller space of $S$. It's well known that $Teich(S)$ is diffeomorphic to a (6g-6)-dimensional cell, where a coordinatization is given by the Fenchel-Nielsen length-twist coordinates $\{(\ell_a, \tau_a) \}_{a\in P}$ associated to a pant decomposition $P$ of $S$.
Question: can we replace the twist parameters with length parameters of other curves, and thereby replace the "length-twist" coordinates with "length-length" coordinates on $Teich(S)$?
Related question: are any formulas known which express the twist differentials $d\tau_a$ with linear combinations of length differentials $d\ell_{a'}$ in Wolpert's formula for the Weil-Petersson symplectic form $\omega_{WP}=\sum_{a\in P} d\ell_a \wedge d \tau_a$ ? I.e. can we express $\omega_{WP}$ using only differentials of length functions?
The same question: if we are given a hyperbolic surface $S'$ with known length parameters relative to a pant decomposition, then what metric properties on the surface $S'$ do we use to identify the twist parameters $\{\tau_a\}_a$?
Remark. This question risks being a duplicate. However we find the answer to the above question unsatisfactory, as indicated by our comments below.
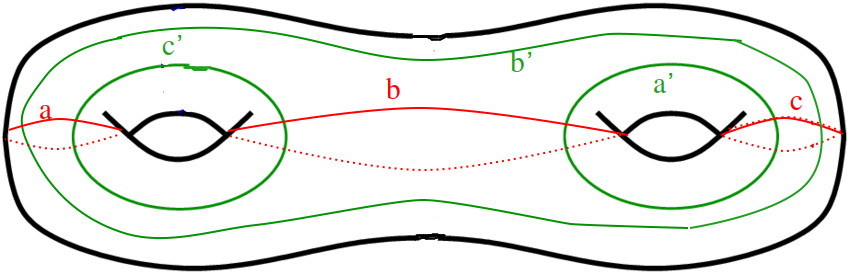
In genus $g=2$ we obtain the following almost canonical collection of six simple closed curves on the surface $S$. The value of the lengths of the green curves do not distinguish between left and right Nielsen twists along the red curves. However the derivatives of the lengths of the green curves do distinguish between left and right Nielsen twists along the red curves. This is similar to how the derivatives of strictly convex functions $f: \mathbb{R}^3 \to \mathbb{R}$ are injective where $Df(x_1)=Df(x_2)$ if and only if $x_1=x_2$. Here we are assuming that the lengths of the green curves are basically convex functions in the Neilsen twist parameters in the red curves.
Answer: Consider genus $g=2$. Let $t(a)$, $t(b)$, $t(c)$ be the Nielsen tangent vectors in Teich defined by the red geodesic pant decomposition $\{a,b,c\}$. Let $\{a', b', c'\}$ be the "dual pant". Then I propose that the functions $$\ell_a,~~~~~ \ell_b,~~~~~ \ell_c$$ together with the ``cosines of the angles of intersection" $$d\ell_{b'}(t(a)),~~~~~ d\ell_{c'}( t(b))~~~~~~,d\ell_{a'}(t(c)))$$ are globally well defined coordinates on Teich. Notice the collection is of cardinality $6g-6=6$ for genus $g=2$. So they are not length-length coordinates, but length-"d"length coordinates. Here I'm assuming all of Wolpert's work, especially pp.252 in Wolpert's 1983 paper referenced by Alex Nolte's answer.
Likewise if we use Wolpert's Reciprocity formula $d \ell_a (t(b))=-d\ell_b(t(a))$, then we obtain another "reciprocal" global coordinate system on Teich. The idea is that the angles of geodesic intersection are effective parameters of the Nielsen twist parameter along the pant cuffs.