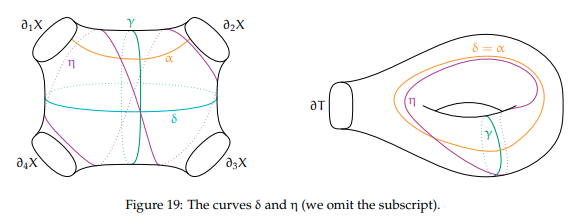
Regarding your second question, explicit expressions are known for the absolute value of the twist parameter $\tau_a$ in term of the lengths $\ell_a$ supplemented with the lengths $\ell'_a$ of certain transverse curves. See for instance equation (5.12) in Andersen, Borot, Charbonnier, Giacchetto, Lewański, Wheeler. On the Kontsevich geometry of the combinatorial Teichmüller space. arXiv:2010.11806. In the case that $\ell_a$, $\tau_a$ are coordinates associated to a curve $\gamma$ that separates two distinct pairs of pants, it allows to solve for $\cosh \tau_a$ in terms of the length $\ell_a$ of $\gamma$, the length $\ell'_a$ of $\delta$ and the lengthlengths of the other four boundaries of the pairs of pants. See Figure 19 in their paper: