Minimal surface enclosing balls

Question

(This question is tangentially related to an earlier question I posed: Minimal surface enclosing two congruent balls.)

Let $B_1,\ldots,B_k$ be unit-radius balls in $\mathbb{R}^3$, with pairwise disjoint interiors. Let $S$ be the minimal area surface that (a) encloses $B_1,\ldots,B_k$ and (b) is topologically a sphere. Then:

Q. Is it the case that never is $S$ the convex hull of $B_1,\ldots,B_k$?

It may be difficult to specify the exact structure of the minimal surface, but can we at least prove that it always "dents inward," and so is never the convex hull?

The "is topologically a sphere" condition requires the balls to be relatively closely packed. Otherwise $S$ might fracture into several components.

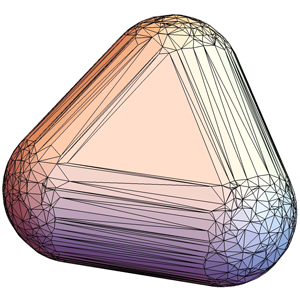
This image from Wrapping juggling balls illustrates the convex hull of three congruent balls:

---

       

---

Answer 1

Here is a proposed argument: Consider the convex hull of the centers of the balls. This is a polyhedron, and the convex hall of the balls consists of all points within radius 1 of it. Take an edge of that polyhedron. The points of radius 1 from that edge form a cylinder, and an open subset of that cylinder is contained in $S$.

A cylinder certainly does not have mean curvature zero. So it is not a minimal surface, and thus has some local deformation shrinking the area, which can be made arbitrarily small. Deforming locally a small amount, the surface will still enclose the balls (since the cylindrical region is not the region touching the balls), proving that $S$ does not have minimum area.