Minimal surface enclosing two congruent balls

Question

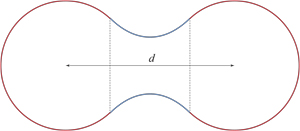
Let $B_1$ and $B_2$ be two unit-radius balls in $\mathbb{R}^3$ whose centers are separated by a distance $d \ge 2$.

Q. For sufficiently small $d$, is the minimal area surface enclosing $B_1$ and $B_2$ formed by spherical caps joined to a catenoid of revolution?

If $d$ is large enough, the minimal surface is just two disjoint spheres. If $d$ is small, then it seems natural that there is a circular ring on the surface of each ball at which a catenoid is tangent, as illustrated in a 2D profile below. Is this known to be the minimal surface? If so, is there a calculation for the position of the rings?

---

      

---

^{See also the related question: Minimal surface enclosing balls.}

Answer 1

This is (mostly) just an answer to your final question. To piece together the surface of the ball with the catenoid, we have to satisfy the following relations, where $\pm x$ denote the horizontal coordinates of the dotted lines in your figure, counted from the midpoint between the balls, and $c$ is the integration constant in the catenoid solution $r(z)=c\cosh (z/c)$: $$ \sqrt{1-(d/2 -x)^2 } = c\cosh (x/c) $$ $$ \frac{(d/2 -x)}{\sqrt{1-(d/2 -x)^2 } } = \sinh (x/c) $$ These two conditions fix $c$ and $x$; plotting $x$ as a function of $d$ (I'm allowing the balls to penetrate one another and am thus plotting starting at $d=0$),

There ceases to be a solution at about $d=2.399357285$. I have checked that this corresponds to the usual point where the ratio of $x$ to the ring radius goes outside the bounds within which a catenoid solution exists. However, already before reaching that maximal value of $d$, the area of the catenoid solution begins to exceed the area of the disjoint sphere solution. This happens at $d=2.319947$. Nonetheless, we therefore see that there is an interval in $d$ above $d=2$ where the catenoid solution has smaller area than the disjoint sphere solution.