Natural boundary with non-zero "thickness"

Question

Many series (in fact, "most" Taylor series) have a natural boundary at the unit circle. This boundary is only 1 dimensional, in the sense that only along the unit circle is it true that there is a dense set of singularities around every point. Do there exist any nice examples of series that have a 2-dimensional space of natural boundaries?

The best I've been able to come up with so far is something like $$\sum_{n=1}^\infty \frac{x^n}{\frac{n}{\alpha} - \beta-x^n} $$ In the complex plane, this looks like:

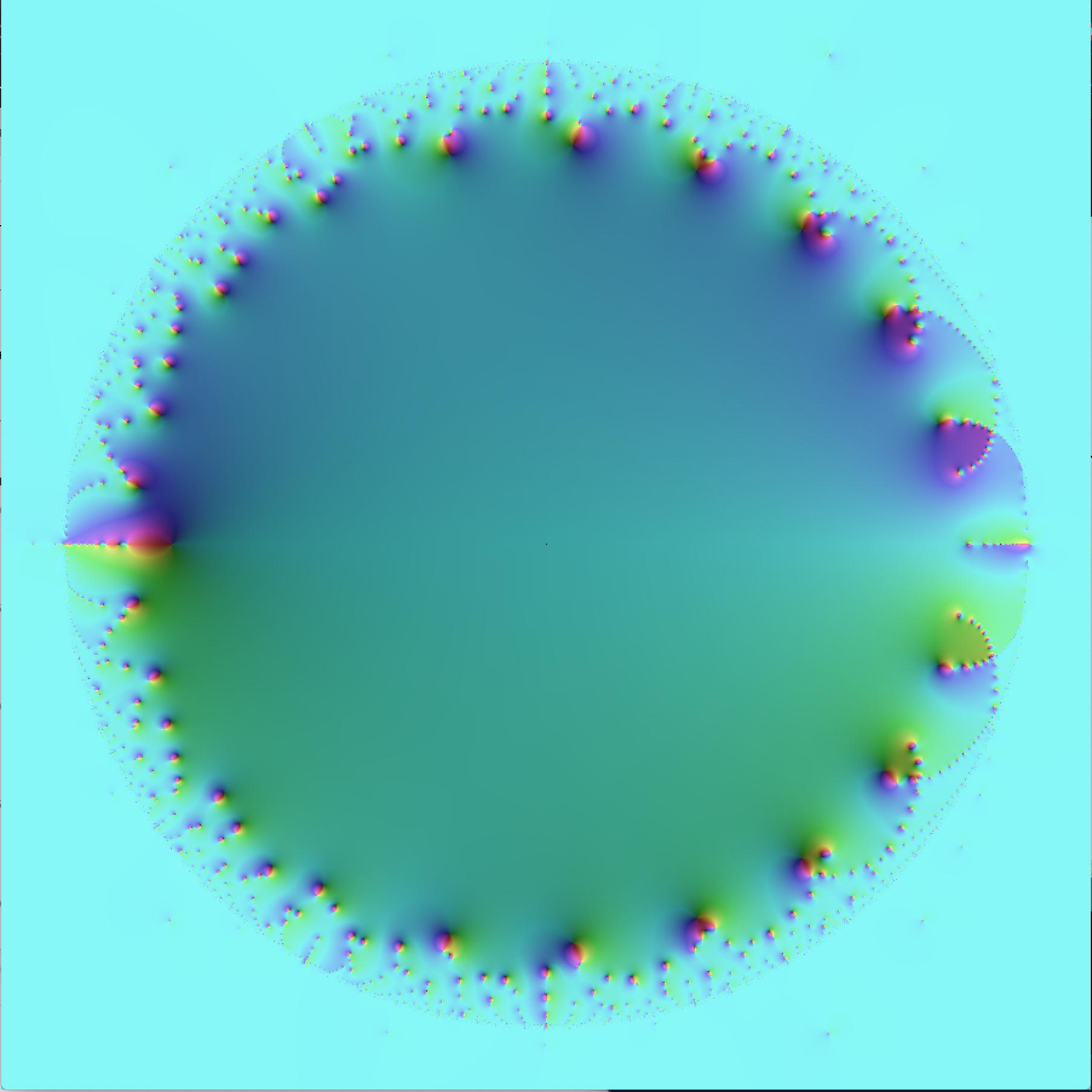
This is close to a solution, since it has many singularities outside of just a circle, however, these singularities don't become dense except along a circle.

I'm thinking there might be some way to craft a function like this by doing something along the lines of: $$\sum_{n=1}^\infty \frac{1}{n^2(\sin(f(x,n)) - \cos(g(x,n)))} $$ However, I can't think of a good way to use this to create dense singularities in some places, and not end up having singularities everywhere.

Any help or ideas would be appreciated!

Answer 1

Existence. (Maybe not a "nice example" as requested, though.)
Take any simple closed curve $S$ with Hausdorff dimension $2$. (I am assuming you mean Hausdorff dimension when you say "dimension".) Take any function $F(z)$ on the unit disk with the unit circle $T$ as its natural boundary. By the Riemann mapping theorem, we get a conformal equivalence $\varphi$ from the region inside $S$ onto the unit disk.
Our new function is $G(z) = F(\varphi(z))$.

Answer 2

It is easy to prove that for every region $D$ there exists a function $f$ analytic in $D$ such that $\partial D$ is the "natural boundary" that is $f$ does not have an analytic continuation into any larger region. So whatever you mean by ``$\partial D$ is $2$-dimensional'', you can always have such an example. Such function is easy to construct: zeros of an analytic function can be arbitrarily assigned, so choose a sequence of zeros whose limit set is dense in $\partial D$.

Another even simpler method is to take a dense countable set $\{ z_k\}$ in $\partial D$ and construct a series $$\sum_k\frac{c_k}{z-z_k}$$ where $c_k$ are so small that the series converges on every compact subset of $D$.