Quadratic cusp shape

Question

Which hyperbolic $3$-manifolds are known to have quadratic cusp shape?

Explanations: Cusps of hyperbolic $3$-manifolds are products torus x interval. They lift to horoballs in hyperbolic $3$-space, where the boundaries (horospheres) inherit a euclidean metric. (Differently sized horoballs yield the same similarity class of Euclidean metric.) The horosphere can thus be identified with ${\bf R}^2$ and projection from the horosphere to the boundary torus of a cusp corresponds to dividing out a lattice from ${\bf R}^2$. The „cusp shape“ is meant to be the shape of this lattice. The question is, for which (say $1$-cusped) manifolds one obtains a square lattice.

Answer 1

The complete list is unknown. In general, it is difficult to determine which manifolds have a prescribed cusp field.

However, there are a well-studied set of examples that namely arithmetic manifolds with (invariant) trace field that is quadratic imaginary. Let me focus first on the manifolds with invariant trace field $Q(i)$. These manifolds are commensurable with the Whitehead link complement and the Borromean rings complement. All of the examples listed in Sam Nead's answer fit this criterion. There are of course more manifolds of this type (as it is an infinite class).

In terms of computed examples, arithmetic data associated to 3-manifolds was studied by

Goodman, Oliver; Heard, Damian; Hodgson, Craig, Commensurators of cusped hyperbolic manifolds, Exp. Math. 17, No. 3, 283-306 (2008). ZBL1338.57016. (and

Coulson, David; Goodman, Oliver A.; Hodgson, Craig D.; Neumann, Walter D., Computing arithmetic invariants of 3-manifolds, Exp. Math. 9, No. 1, 127-152 (2000). ZBL1002.57044.). The data computed in the first reference is more relevant here, it is accessible on Craig Hodgson's webpage: https://researchers.ms.unimelb.edu.au/~snap/commens.html

Also, the tables of data at the end of:

Maclachlan, Colin; Reid, Alan W., The arithmetic of hyperbolic 3-manifolds, Graduate Texts in Mathematics. 219. New York, NY: Springer. xiii, 463 p. (2003). ZBL1025.57001.

Finally, there is a knot complement $S^3 \setminus 12n706$ often referred to as the Boyd knot complement (for David Boyd) is a knot complement which is not commensurable with the Whitehead link complement but has cusp field $Q(i)$. The knot complement decomposes into regular ideal tetrahedra and octahedra and is beautiful in its own right. Attached is a picture of the cusp neighborhood computed by SnapPy.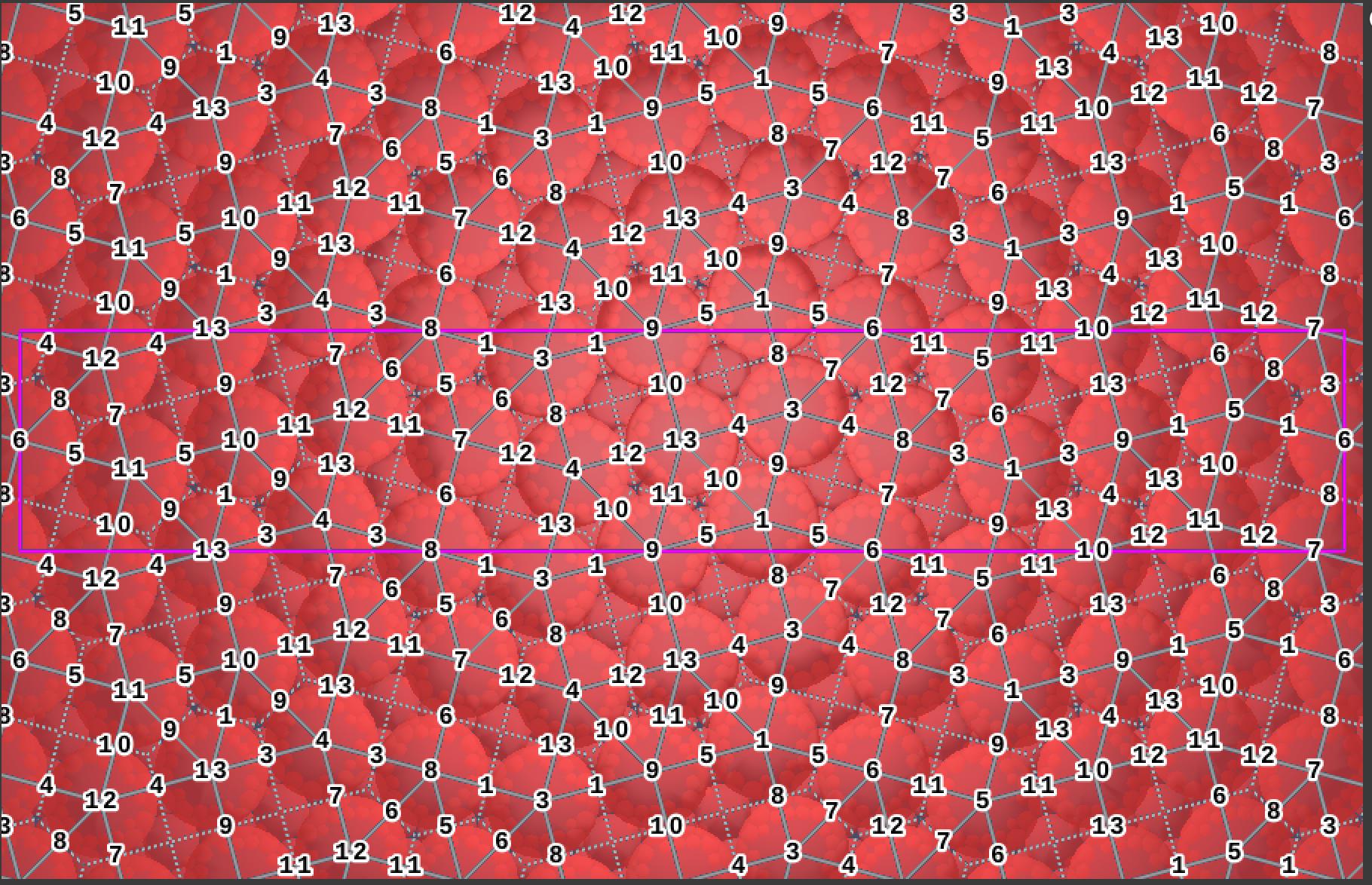

In terms of quadratic cusp shape, there are three other knot complements known to have this property, but those cusp shapes are in $Q(\sqrt{-3})$. One is the figure eight knot complement and the other two are the dodecahedral knot complements of Aitchison and Rubinstein:

Aitchison, I. R.; Rubinstein, J. H., Combinatorial cubings, cusps, and the dodecahedral knots, Topology ’90, Contrib. Res. Semester Low Dimensional Topol., Columbus/OH (USA) 1990, Ohio State Univ. Math. Res. Inst. Publ. 1, 17-26 (1992). ZBL0773.57010. 1: https://i.sstatic.net/DE0Aa.jpg

Answer 2

Here is a quick search using snappy:

In[29]: C = OrientableCuspedCensus(num_cusps = 1)

In[30]: square = [M.name() for M in C if abs(M.cusp_info()[0].modulus - 1j) < 0.00002]; square

Out[30]: ['m130', 'm135', 'm139', 'v1859', 'v3318', 't07829', 't12033', 't12035', 't12036', 't12038', 't12040', 't12041', 't12043', 't12045', 't12050', 'o9_17193', 'o9_19556', 'o9_21441', 'o9_22828', 'o9_31519', 'o9_31521', 'o9_35959', 'o9_41335', 'o9_42724']

A couple of the t's are double covers of the m's. I'll guess that it is not too hard to construct manifolds with square cusp. Finally, I don't see why there should be any particular pattern to these manifolds (beyond some covering relations, sometimes).

EDIT:

I vaguely recall that Craig Hodgson (Melbourne) showed me at some point a list of hyperbolic manifolds with particularly nice cusp shapes, including square and hexagonal. I believe that his list was made by trawling the snappy census, but perhaps he has further thoughts about these manifolds.