I need a function $f(x)$ with the following properties -
- It should be monotonically non-decreasing.
- For $x \geq 1$, $x + \frac{1}{x} - f(x) < \epsilon$ where $\epsilon$ is an extremely small positive real number.
- It should look simple. I know this sounds like a very vague requirement, but I hope the meaning of "simple" is clear to some extent. For example, the function should definitely not have a piecewise definition. One should be able to write it by using not more than 15 characters, etc. etc.
Can anyone think of such a function? It's fine even if the above requirements are satisfied only for positive values of $x$.

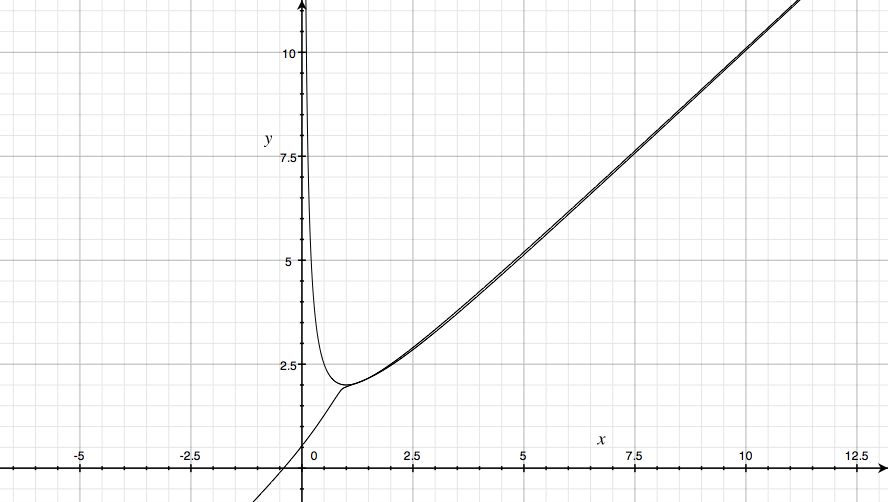
$(0,\infty)$as required. $\endgroup$