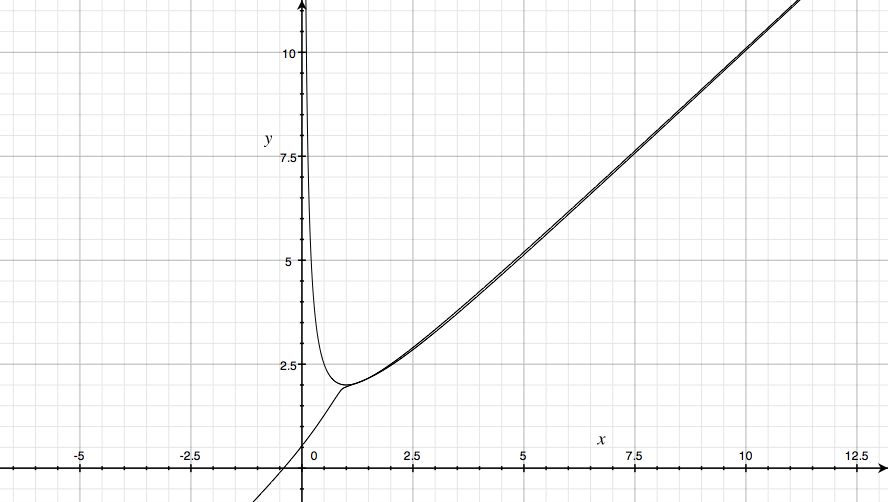
This may be more than 15 symbols, but $$ f(x)= x + \frac{1}{1+(x+\delta-1)^{2n/(2n-1)}} $$ where $\delta>0$ is small and $n$ is large. For $n=2$ and $\delta=1/10$ it looks like this:
 alt text http://www.math.hawaii.edu/%7Ebjoern/Teaching/MO.jpg(source)
alt text http://www.math.hawaii.edu/%7Ebjoern/Teaching/MO.jpg(source)
EDIT:
Motivation
The idea is that we want $y=x+g(x)$ where $g$ is a bell curve of some sort, say $g(x)=e^{-x^2}$. Something that is a little more reminiscent of $1/x$ is $$ g_{1,1}(x)=\frac{1}{1+x^2} $$ but the $x^2$ term means that the rate of approach to $1/x$ is not so good. On the other hand $$ g_{\infty,0}(x)=\frac{1}{1+|x-1|} $$ would be perfect, were it differentiable. The formula $$ g_{n,\delta}(x)= \frac{1}{1+(x+\delta-1)^{2n/(2n-1)}} $$ gives something that looks like the latter from far away ($n=\infty$, $\delta=0$), and like the former from up close ($n=\delta=1$). Note that $|a|=(a^2)^{1/2}$.
EDIT #2:
Or...
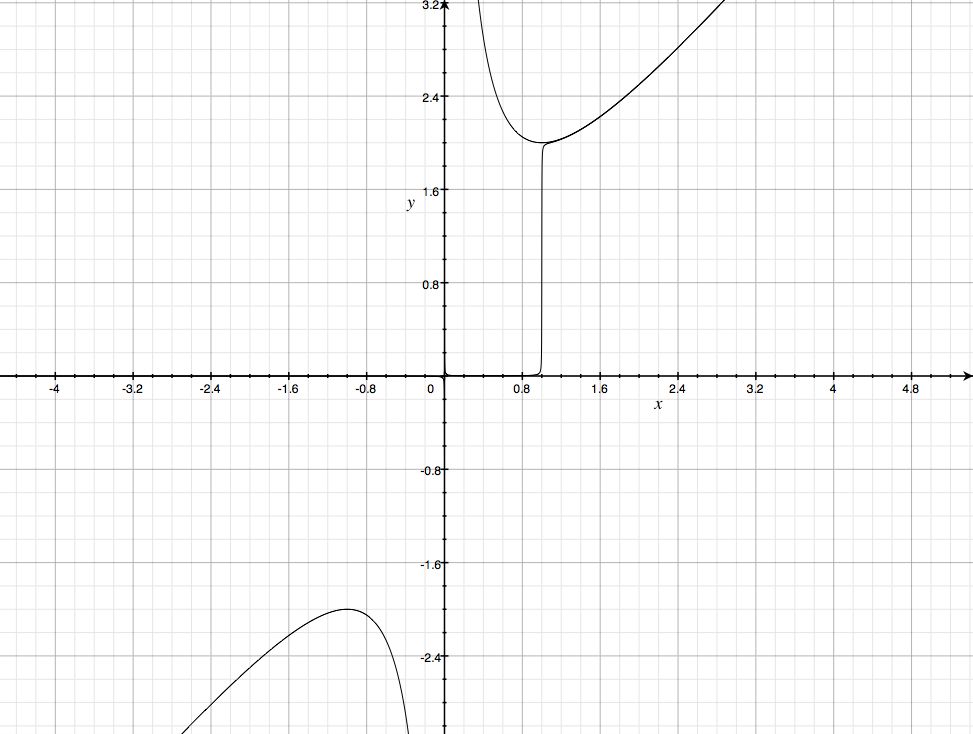
Here is a plot of Gowers' more general solution (in another answer to this question), for this specific case, together with the original curve:
 alt text http://www.math.hawaii.edu/%7Ebjoern/Teaching/Gowers.jpg(source)
alt text http://www.math.hawaii.edu/%7Ebjoern/Teaching/Gowers.jpg(source)
