2
$\begingroup$

Let $f\in L^p(\mathbb{R})$ and define $f_\theta(x)=f(x-\theta)$. Let $K\subset\mathbb{R}$ be a compact set. I would like to compute (or at least lower bound) the following: $$ \inf_{\theta\ne\theta'\in K}\frac{\Vert f_\theta - f_{\theta'}\Vert_p}{|\theta-\theta'|}. $$ In particular, I want to understand how this depends on $f$, and would like a bound that depends explicitly on $f$. This is also where the properties of $f$ come in: The weaker the assumptions the better, but e.g. if there a nice bound that depends (say) on the deriviatives of $f$, then we can assume the needed regularity.

My suspicion is that there is an easy counterexample to show this can be rather poorly behaved even for smooth functions, but I have not been creative enough so far.

Improve this question
$\endgroup$
3
  • $\begingroup$ Assume $|f(x) - f(y)| \geq L |x-y|$ uniformly then $|f_{\theta}(x) - f_{\theta'}(x)| = |f(x-\theta) - f(x-\theta')| \geq L|\theta - \theta'|. $ In particular, $\|f_{\theta}- f_{\theta'} \|_p \geq L|\theta - \theta'| diam(K)^{1/p}$. So a lower bound would be $L diam(K)^{1/p}$. I don't know how useful this is for you as it pretty much limits $f$ to being strictly monotone with derivative uniformly bounded from $0$. $\endgroup$
    – Lars
    Commented Jul 22, 2021 at 21:34
  • $\begingroup$ For the above, I took $\|\cdot\|_p$ as the norm in $L^p(K)$, but you might not want this. $\endgroup$
    – Lars
    Commented Jul 22, 2021 at 22:04
  • $\begingroup$ @LarsvanderLaan : There is no function $f\in L^p(\mathbb R)$ for $p>0$ such that $|f(x)-f(y)|\ge L|x-y|$ for some real $L>0$ and all real $x,y$. $\endgroup$
    – Iosif Pinelis
    Commented Jul 23, 2021 at 15:07

1 Answer 1

Reset to default
1
$\begingroup$

Here "is an easy counterexample to show this can be rather poorly behaved even for smooth functions". The idea is to make $f$ almost periodic with a quasi-period $T$ (but with a slowly decreasing amplitude near $\pm\infty$, to satisfy the condition $f\in L^p(\mathbb R)$), and then shift $f$ by $T$. Then the shifted function $f_T$ will differ little from $f_0=f$.

For instance, let $$f(x):=c(1+\sin x)e^{-c^2 (x-\pi)^2},$$ where $c>0$ is small. Then $\|f\|_1=\sqrt\pi$ for all real $c>0$. However, $$\frac{\|f_{2\pi}-f_0\|_1}{2\pi}=\frac{\text{erf}(c\pi)}{\sqrt\pi}\to0$$ as $c\downarrow0$. So, there is no nontrivial (that is, nonzero) lower bound here -- unless $f$ is fixed or, more, generally, other conditions on $f$ are imposed, in addition to smoothness; see below.

Here is the graph $\{(x,f(x))\colon|x|<11\pi\}$ for $c=1/100$:

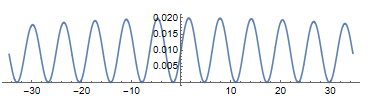
enter image description here

$\newcommand\de\delta$Now a simple result in the positive direction. Suppose that for some real $c>0$ and all $x\in[a,b]$ we have $f'(x)\ge c$. Then for any real $\de>0$ and all $x\in[a,b-\de]$ we have $f(x+\de)-f(x)\ge c\de>0$ and hence $\|f_\de-f_0\|_p\ge c\de(b-a-\de)_+^{1/p}$, where $u_+:=\max(0,u)$.

Suppose now that (i) for some real $c_1>0$ and all $x\in[a_1,b_1]$ we have $f'(x)\ge c_1$ and (ii) for some real $c_2>0$ and all $x\in[a_2,b_2]$ we have $f'(x)\le -c_2$. Then, similarly, for any real $\de>0$ we have $\|f_\de-f_0\|_p^p\ge c_1^p\de^p(b_1-a_1-\de)_+ + c_2^p\de^p(b_2-a_2-\de)_+$. So, if $K-K\subset[-\de_*,\de_*]$ for some real $\de_*>0$, then the infimum in question is $$\ge(c_1^p(b_1-a_1-2\de_*)_+ + c_2^p(b_2-a_2-2\de_*)_+)^{1/p}.$$

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks for the detailed answer! I am not sure about one thing, though: How do you deduce that "there is no nontrivial (that is, nonzero) lower bound here"? Perhaps I am misunderstanding your argument, but if you need to take $c\to 0$, then $f$ is no longer fixed. So there is no nontrivial lower bound for all $c>0$, but for any fixed $c>0$ (and hence fixed $f$), such a lower bound could still exist. $\endgroup$
    – tim622
    Commented Jul 23, 2021 at 17:23
  • $\begingroup$ @tim622 : Indeed, to get a nonzero lower bound, we need to fix $f$ or impose additional conditions on it (I have now added this comment to the answer). One way to go in the positive direction is shown in the second part of the answer. $\endgroup$
    – Iosif Pinelis
    Commented Jul 23, 2021 at 17:46
  • $\begingroup$ Perfect, thanks for clarifying. One more question: In your positive direction, should "for any real $\delta>0$" be "for all sufficiently small $\delta>0$"? $\endgroup$
    – tim622
    Commented Jul 23, 2021 at 18:15
  • $\begingroup$ @tim622 : These two variants would mean the same in this context. Indeed, if $\delta>b-a$, then the interval $[a,b-\delta]$ is empty and $(b-a-\delta)_+=0$. $\endgroup$
    – Iosif Pinelis
    Commented Jul 23, 2021 at 18:20

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .