Mutual Information of the summation of a Chi-square random variable and a Gaussian variable

Question

As the title, $X$ is an random variable subject to $N(0,1)$, $N$ is an random variable subject to $N(0,\sigma^2)$, and $X$ and $N$ are independent. I want to calculate the mutual information $I(X,Y)$ between $X$ and $Y=X^2+N$. The problem can be reduced to calculate the differential entropy $h(Y)$ of $Y$. However, $h(Y)=-\int_{-\infty}^{+\infty}p_Y(y)log(p_Y(y))\mathrm{d}y$, and the $p_Y(y)$ can not get the explicit form. How can I calculate $h(Y)$ or $I(X,Y)$ can be calculated in other ways?

Answer 1

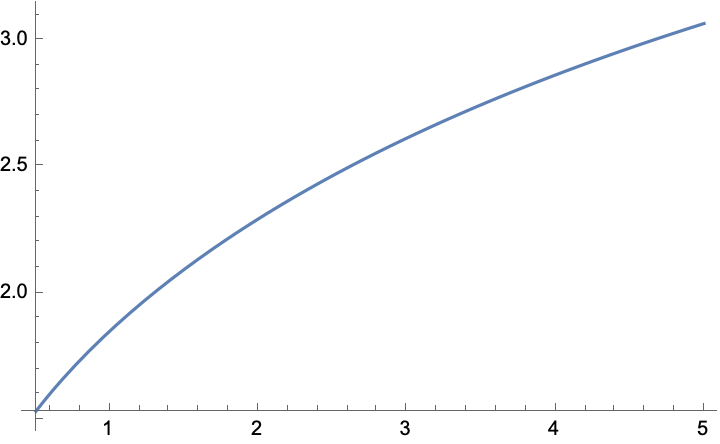
It is not quite true that $p_Y(y)$ has no explicit form, it is given by $$p_Y(y)=\int_0^\infty \frac{e^{-(w-y)^2/2 \sigma^2-w/2}}{2 \pi \sigma \sqrt{w}}\,dw=f_1(y)\theta(2y-\sigma^2)+f_2(y)\theta(\sigma^2-2y),$$ with $\theta(y)$ the unit step function and $$f_1(y)=\frac{1}{4\sigma\sqrt 2}e^{\frac{\sigma^4-4 \sigma^2 y-4 y^2}{16 \sigma^2}} \sqrt{2y-\sigma^2} \left[I_{1/4}\left(\frac{\left(\sigma^2-2 y\right)^2}{16 \sigma^2}\right)+I_{-1/4}\left(\frac{\left(\sigma^2-2 y\right)^2}{16 \sigma^2}\right)\right],$$ $$f_2(y)=\frac{1}{4\pi\sigma}e^{\frac{\sigma^4-4 \sigma^2 y-4 y^2}{16 \sigma^2}} \sqrt{\sigma^2-2 y}\; K_{1/4}\left(\frac{\left(\sigma^2-2 y\right)^2}{16 \sigma^2}\right).$$ The differential entropy $h(Y)$ can be readily evaluated numerically, here is a plot of $h(Y)$ versus $\sigma$.