I have 2 IID random variables $X_1$ and $X_2$ with $Bern(p)$ distribution. I have another binary random variable $Y$ taking values in $\{0,1\}$.
I am interested in comparing the following 2 mutual information $I(X_1+X_2;Y)$ and $I(2X_1;Y)$. Note that $Y=0$ with probability $\frac{1}{x+5}$ when the input ($X_1+X_2$ or $2X_1$) takes the value $x$.
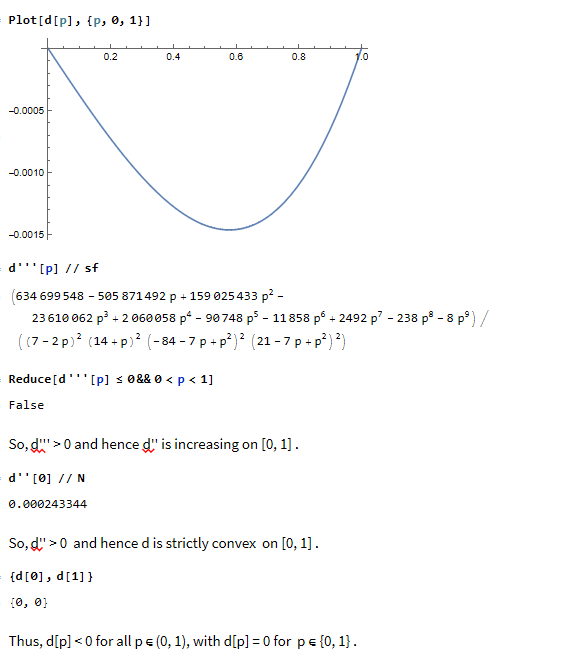
I have a feeling that $I(X_1+X_2;Y) \leq I(2X_1;Y)$. Can someone help me prove or disprove this?