Assume $\mathbf{x}$ is a random vector with mean $\mathbf{\mu}$ and covariance matrix $\mathbf{\Sigma}$. Symmetric matrices $\mathbf{A}$ and $\mathbf{B}$ are given.
Without assuming normality, how to prove that the covariance matrix of the quadratic form is the following?
$$ \mbox{Cov} \left( \mathbf{x'Ax,x'Bx} \right) = 2 \mbox{Tr} \left( \mathbf{A\Sigma B\Sigma} \right) + 4 \mathbf{\mu^T A\Sigma B \mu} $$
I found a proof but assuming normality. Can anyone please give me some hint or reference to prove this? Thanks a lot!
I found Robert's suggestion pretty reasonable. I found this question is taken from the book 《Modern multivariate analysis》, Casella.
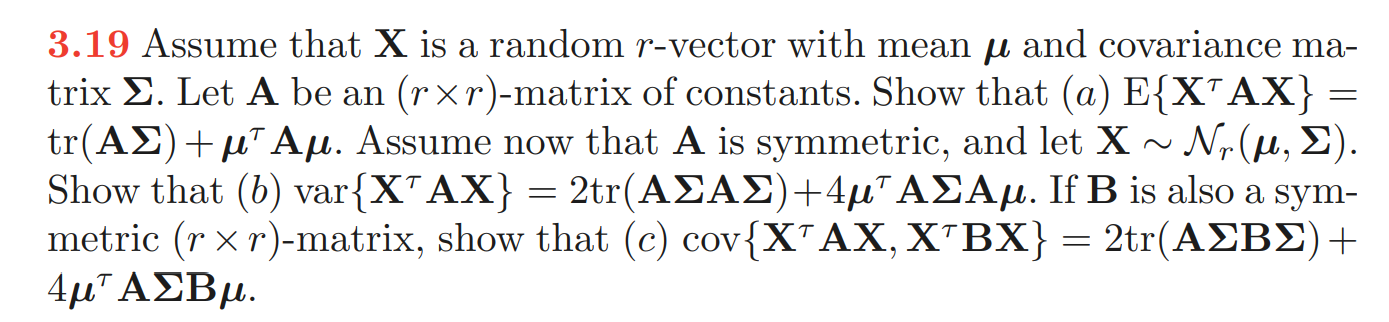
It's right to assume normality.
