A generalization of Harcourt's theorem

Question

This question is closely related to my previous question.

Can you prove the claim given below? The following claim is a conjectured generalization of Harcourt's theorem.

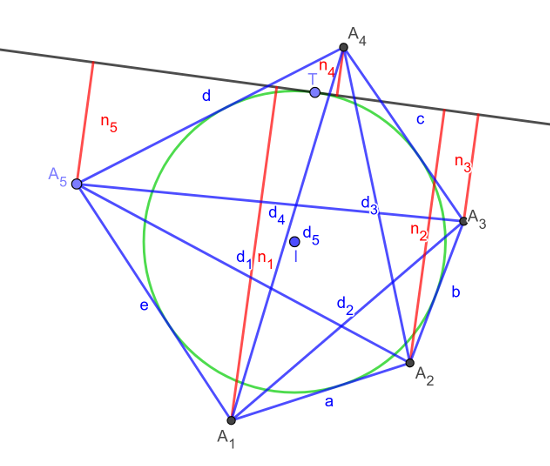
Claim. Let $A_1,A_2 \ldots A_n$ be the vertices of $n$-sided bicentric polygon with semiperimeter $s$ and area $K$. Let $d_i$ be the length of the line segment whose endpoints are polygon's vertices adjacent to the vertex $A_i$ . Let line $t$ be the tangent to the polygon's incircle at any point on that circle. Denote the signed perpendicular distance of the vertex $A_i$ from the tangent line as $n_i$ , with a distance being negative if and only if the vertex is on the opposite side of the tangent line from the incenter. Then, $$K=\frac{s \cdot \displaystyle\sum_{i=1}^n n_i \cdot d_i}{\displaystyle\sum_{i=1}^n d_i}$$

Picture for the case $n=5$:

GeoGebra applets that demonstrate this claim in the case of $n=5$ and $n=6$: Area of bicentric pentagon , Area of bicentric hexagon. The proofs for the case $n=3$ and the case $n=4$ can be found here and here ,respectively.

Answer 1

So we need to show $r\cdot \sum d_i=\sum n_id_i$, where $r$ denotes the inradius. Rewrite this as $0=\sum (n_i-r)d_i$, denote $n_i-r=m_i$. Then $m_i=:f(A_i)$ is the signed distance from $A_i$ to the line passing through $I$ and parallel to $t$. I claim $$\sum d_iA_i=(\sum d_i)I,\quad\quad\quad\quad(\heartsuit)$$ this yields $0=(\sum d_i)f(I)=f(\sum d_iA_i)=\sum d_if(A_i)=\sum d_im_i$.

Note that $d_i=2R\sin \angle A_{i-1}A_iA_{i+1}$ where $R$ is the circumradius. Denote by $B_i$ the tangency point of $A_iA_{i+1}$ and the incircle. Then $IB_{i-1}A_iB_i$ is a cyclic quadrilateral with diameter $IA_i$, thus $B_{i-1}B_i=IA_i\cdot \sin \angle A_{i-1}A_iA_{i+1}$, and the vector $(A_i-I)\cdot \sin \angle A_{i-1}A_iA_{i+1}$ is obtained from $B_i-B_{i-1}$ by a rotation on angle $\pi/2$ (clockwise on your picture). Since the sum of vectors $B_i-B_{i-1}$ is zero, the sum of rotated vectors is also 0, as needed.