Geometry interpretation of any continuous random variable

Question

Given a continuous random variable $X$ with the cdf $F_X(x)$, I want to know whether there exists a random vector $\mathbf{Z}$ uniformly distributed in a geometry region $\mathscr{Z}_n$ in $\mathbb{R}^n$ such that each one-dimensional marginal distribution $F_{\mathscr{Z}_n}^1$ of $\mathbf{Z}$ is close to the distribution of $X$. In addition, an asymptotic perspective is also welcome. For example, it is also meaningful to know whether $F_{\mathscr{Z}_n}^1=F_X$ is possible if $n$ tends to $+\infty$.

---

Some useful facts are given as follows:

1. A normal distribution can be approximated by a uniform random variable distributed in a hypersphere.
2. A negative exponential distribution can be approximated by a uniform random variable distributed in a simplex.

---

This question has been asked in Mathematics Stack Exchange yesterday.

Answer 1

$\newcommand\ep\epsilon\newcommand\de\delta\newcommand\1{\mathbf1}\newcommand\R{\mathbb R}\newcommand\U{\mathscr U}$Previously, I suggested that for any probability distribution $\mu$ over $\R$ there exists a subset $A$ of $\R^n$ of nonzero Lebesgue measure such that each one-dimensional marginal of the uniform distribution $\U_A$ over $A$ is exactly $\mu$. I now doubt that.

However, we can show the following: For each natural $n$ and each probability distribution $\mu$ over $\R$ there is a family $(A^\ep\colon\ep>0)$ of subsets $A^\ep$ of $\R^n$ of nonzero Lebesgue measure such that each one-dimensional marginal of the uniform distribution $\U_{A^\ep}$ over $A^\ep$ converges (weakly) to $\mu$ (as $\ep\downarrow0$).

Convolving $\mu$ with a distribution with a bounded continuous mollifier density (with respect to the Lebesgue measure over $\R$), we see that without loss of generality (wlog) $\mu$ has a bounded continuous density $p$. Let the desired set $A^\ep$ be of the form \begin{equation*} A^\ep:=\{x\in\R^n\colon \|x-(x\cdot\1)\1/n\|_n\le\ep\, p(x\cdot\1/n)^{1/(n-1)}\}, \tag{1} \end{equation*} where $\cdot$ is the dot product over $\R^n$, $\1:=(1,\dots,1)\in\R^n$, and $\|\cdot\|_n$ is the Euclidean norm on $\R^n$.

A more geometric description of the set $A^\ep$ is as follows. Consider
the diagonal \begin{equation*} D:=\{t\1\colon t\in\R\}. \end{equation*} Let $\Pi_t$ denote the affine hyperplane through the point $t\1$ perpendicular to the diagonal line $D$; let us refer to the point $t\1$ as the origin of the affine hyperplane $\Pi_t$. For each $x\in\R^n$ there is a unique real $t_x$ such that $x\in\Pi_{t_x}$; actually, $t_x=x\cdot\1/n$, the arithmetic mean of the coordinates of $x$. Then $A^\ep$ is the set of all points $x\in\R^n$ whose (shortest) (Euclidean) distance to the diagonal $D$ is $\le\ep\,p(t_x)^{1/(n-1)}$.

Now take any real $a$. Let \begin{equation*} V_a:=\{(x_1,x_2,\dots,x_n)\in\R^n\colon x_1=a\}, \end{equation*} the "vertical" $(n-1)$-dimensional affine hyperplane consisting of all points in $\R^n$ with "abscissa" $a$. We want to show that \begin{equation*} \frac{|A^\ep_a|}{\ep^{n-1}}\to c p(a) \tag{2} \end{equation*} for some real $c>0$ (depending only on $n$), where $|A^\ep_a|$ is the Lebesgue measure of the "vertical" $a$-cross-section $$A^\ep_a:=A^\ep\cap V_a$$ of $A^\ep$, consisting of all points in $A^\ep$ with "abscissa" $a$; that is, $|A^\ep_a|$ is the Lebesgue measure of the set $\{(v_2,\dots,v_n)\in \R^{n-1}\colon a\1+(0,v_2,\dots,v_n)\in A^\ep\}$.

Since the density $p$ of $\mu$ is bounded, we have $p\le C^{n-1}$ for some real $C>0$. Since the diagonal $D$ does not lie on the affine hyperplane $V_a$, there is a real constant $b>0$ such that, if the distance of a point $x\in A^\ep_a$ from the point $a\1\in A^\ep_a$ is $>\ep Cb$, then the distance of $x$ from the diagonal $D$ is $>\ep C\ge\sup_s \ep p(s)^{1/(n-1)}$, which implies that $x\notin A^\ep$. (Here, one can take $b=\sqrt n$.) So, the distance of any point $x\in A^\ep_a$ from the point $a\1\in A^\ep_a$ is $\le\ep Cb$, and hence this point $x$ lies on the affine hyperplane $\Pi_t$ for some real $t$ such that $|t-a|\le\ep Cb$. Let now \begin{equation*} m^\ep_a:=\min\{p(t)^{1/(n-1)}\colon |t-a|\le\ep Cb\},\quad M^\ep_a:=\max\{p(t)^{1/(n-1)}\colon |t-a|\le\ep Cb\}. \end{equation*} Then, by the continuity of $p$, \begin{equation*} m^\ep_a\to p(a)^{1/(n-1)},\quad M^\ep_a\to p(a)^{1/(n-1)}. \tag{3} \end{equation*}

Let now $Px$ denote the orthogonal projection of a point $x\in\R^n$ onto the hyperplane $\Pi_0$.

It follows that

(i) if the projection $Px$ of a point $x\in V_a$ is at distance $\le m^\ep_a$ from the origin (of both $\R^n$ and the hyperplane $\Pi_0$), then $x\in A^\ep_a$;

(ii) if the projection $Px$ of a point $x\in V_a$ is at distance $>M^\ep_a$ from the origin, then $x\notin A^\ep_a$.

Thus, for some real constant $c>0$ depending only on $n$, \begin{equation*} c\,(\ep m^\ep_a)^{n-1}\le |A^\ep_a|\le c\,(\ep M^\ep_a)^{n-1}. \end{equation*} So, (2) follows in view of (3).

That is, the density of the first of the $n$ one-dimensional marginals of the uniform distribution $\U_{A^\ep}$ over $A^\ep$ converges pointwise to the density $p$ of $\mu$. So, by Scheffé's lemma, the first of the $n$ one-dimensional marginals of $\U_{A^\ep}$ over $A^\ep$ converges to $\mu$ in total variation. The same holds for each of the $n$ one-dimensional marginals of $\U_{A^\ep}$, since the sets $A^\ep$ are invariant with respect to any permutation of the coordinates. $\Box$

---

This is illustrated by Mathematica' work for $n=2$ and $p(x):=\max(0,1-|x-1|)$ as follows:

---

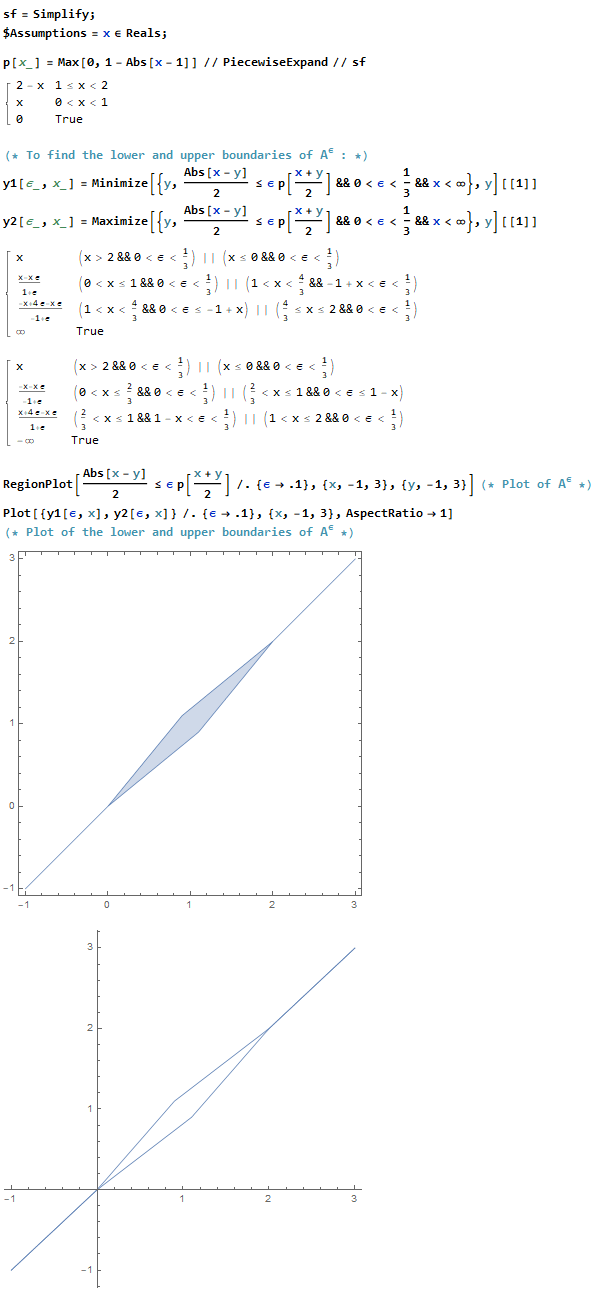
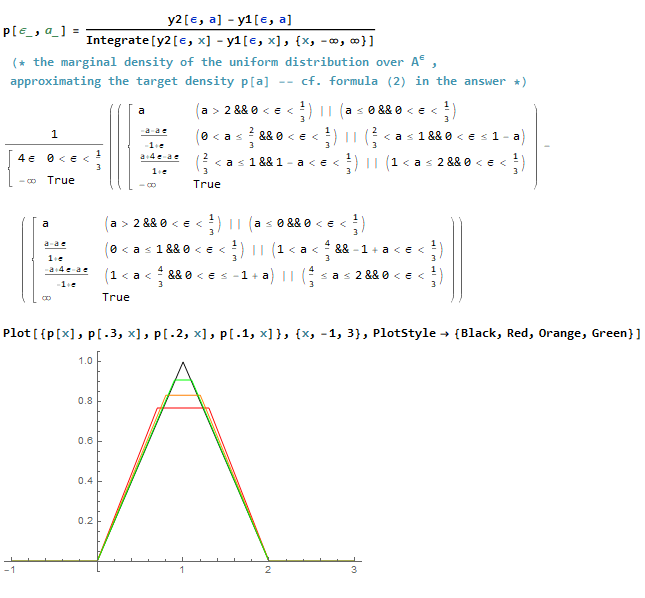
Comment: When writing the first paragraph of this answer, I had in mind the explicit construction (1), which actually would later turn out to be in line with a subsequent comment by the OP about desirable simple topological properties of the region.

As now pointed out in the comment by Paata Ivanishvili, actually for any probability distribution $\mu$ over $\R$ with a bounded density $p$ there does exist a subset $A$ of $\R^n$ of nonzero Lebesgue measure such that each one-dimensional marginal of the uniform distribution $\U_A$ over $A$ is exactly $\mu$.

The idea here is to consider the convex set $F$ of measurable functions $f\colon\R^n\to[0,1]$ such that the one-dimensional marginals of $f(x)\,dx$ are $cp(t)\,dt$ for $c:=1/\|p\|_\infty^n$. The set $F$ is nonempty, because the function $f$ given by $f(x_1,\dots,x_n)\equiv cp(x_1)\cdots p(x_n)$ is in $F$. If now $F$ has an extreme point, it remains to show that any function in $F$ that is an extreme point of $F$ takes only values $1$ or $0$ almost everywhere (which looks very plausible, at least). Indeed, such an extreme point of $F$ coincides almost everywhere with the indicator of a measurable subset $A$ of $\R^n$ of nonzero Lebesgue measure such that each one-dimensional marginal of the uniform distribution $\U_A$ over $A$ is $\mu(dt)=p(t)\,dt$. This idea can of course be generalized to more general marginals.

However, this extreme-point construction is not explicit and the resulting set $A$ may turn out to have poor topological properties. In this respect, the simple and explicit construction (1) may still be of interest, even though it only approximates the marginals and works only when all the one-dimensional marginals are equal to one another. $\Box$

Answer 2

Let $f_X$ be the pdf of $X$. Let $$C = \int_{-\infty}^{\infty} f_X(x) \log F_X(x) dx $$

and define $$\mathscr{Z}_n = \left\{ x_1,\dots, x_n \in \textrm{Supp}(X) \mid \sum_{i=1}^n \log f_X(x_i) \geq n C \right\}$$

Note that this specializes to your examples - for a Gaussian, $\log f_X$ is quadratic, so this defines a ball, and for an exponential distribution, $\log f_X$ is linear (and supported on nonnegative reals) so this defines a simplex.

Let $\mathbf Z$ be uniform on $\mathscr{Z}_n$. If $X$ is uniform on its support $\textrm{Supp}(X)$, then $\mathscr{Z}_n =\textrm{Supp}(X)^n$ and the marginals of $\mathscr{Z}_n$ are all $X$.

Otherwise, the marginal distribution of $\mathscr{Z}_n$ has pdf proportional to

$$\int_{x_2,\dots, x_n \in \textrm{Supp}(X)} A(x_1, x_2,\dots,x_n) dx_2\dots dx_n$$ where $$A(x_1, x_2,\dots,x_n) = \begin{cases} 1 & \sum_{i=1}^n \log f_X(x_i) \geq n C \\ 0 & \sum_{i=1}^n \log f_X(x_i) < n C\end{cases}$$

which is equal to

$$f_X(x_1) \int_{x_2,\dots, x_n \in \mathbb R} B(x_1, x_2,\dots,x_n) f_X(x_2)\dots f_X(x_n) dx_2\dots dx_n$$

where $$B(x_1, x_2,\dots,x_n) = \begin{cases} e^{ n C - \sum_{i=1}^{n} \log f_X(x_i)} & \sum_{i=1}^n \log f_X(x_i) \geq n C \\ 0 & \sum_{i=1}^n \log f_X(x_i) < n C\end{cases}$$

So it suffices to check that the expectation of the random variable $B(x_1,\dots,x_n)$, with $x_2,\dots, x_n$ distributed according to $X$, is independent of $x_1 \in \textrm{Supp}(X)$. In fact, we will check that when multiplied by $\sqrt{n}$, it converges to a constant independent of $x_1$.

In other words, this is the expectation of $$\begin{cases} e^{-y} & y\geq 0 \\ 0 & y <0 \end{cases}$$ where $y = \sum_{i=1}^n \log f_X(x_i) - nC$

By the local central limit theorem, the distribution of $ \sum_{i=2}^n \log f_X(x_i) - (n-1)C$ is approximately a Gaussian with mean $0$ and variance $(n-1) v$ for $v>0$ the variance of $\log f_X(x)$. Thus, multiplied by $\sqrt{n}$, the measure of this random variable converges to a uniform measure on $\mathbb R$. Since $y$ is this random variable plus $x_1$, the expectation of $$\begin{cases} e^{-y} & y\geq 0 \\ 0 & y <0 \end{cases}$$ converges to a constant times $\int_{0}^\infty e^{-y} dy$.

Alternatively, if $\log f_X(x)$ is supported in an arithmetic progression, then the distribution of $ \sum_{i=2}^n \log f_X(x_i) - (n-1)C$ is approximately a Gaussian restricted to that arithmetic progression, so multiplying by $\sqrt{n}$ it converges to the uniform measure on the arithmetic progression, and the expectation converges to a constant times $\sum_{n=0}^{\infty} e^{-qn}$.