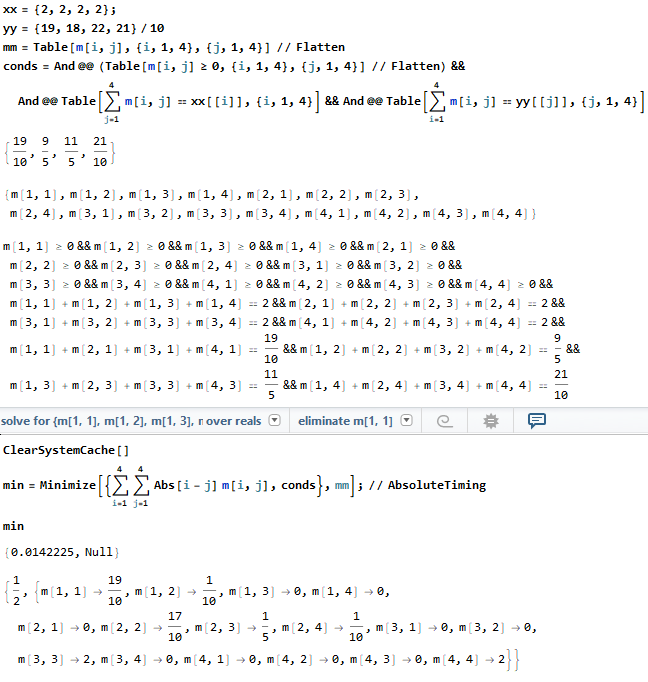
Im trying to construct a distance Measure between two vectors, that takes into account also the distance between the Dimensions. I will illustrate my Problem with some examples:
$x,y \in \mathbb{R}^n$, $d(x,y)$ fullfills the metric-axioms (but im not shure if the triangle-equality must hold - so a semi-metric could also probably do the job).
1.)
$x_1=1,x_2=1,x_3=1,x_4=1$;
$y_1=0,9;y_2=1,1;y_3=1;y_4=1$;
$d(x,y)=0,1 $
In this example 0.1 is moved from $𝑥_1$ to $𝑦_2$, so the distance between the coordinates is 1, times 0.1 gives $d(x,y)=0.1$.
2.) $x_1=1;x_2=1;x_3=1;x_4=1$;
$y_1=0,9;y_2=1;y_3=1;y_4=1,1$;
$d(x,y)=0.3$
In the second case 0.1 is moved from $x_1$ to $𝑦_4$, so the distance between the coordinates is 3 times 0.1 gives $d(x,y)=0.3$.
3.)
$x_1=2;x_2=2;x_3=2;x_4=2$;
$y_1=1,9;y_2=2,1;y_3=1.9;y_4=2,1;$
$d(x,y)=0.2$
In this example 0.1 is moved from 𝑥1 to 𝑦1 equates to 0.1. Also 0.1 is moved from $𝑥_3$ to $𝑦_4$. In total we moved 0.2 mass from $x_i$ to $x_{i+1}$. Equates to $d(x,y)=0.2$
4.)
$x_1=2;x_2=2;x_3=2;x_4=2$;
$y_1=1,9;y_2=1,8;y_3=2,2;y_4=2,1$;
$d(x,y)=0.5$
In this case either 0.1 is moved from $𝑥_1$ to $𝑦_4$ and 0.2 from $𝑥_2$ to $𝑦_3$. Equates to $d(x,y)=0.3+0.2$. Some more explanations are also possible,i.e: 0.1 is moved from $x_1$ to $y_3$ equals to $d_1(x,y)=0.2$. 0.1 are moved from $x_2$ to $y_3$, $d_2(x,y)=0.1$. 0.1 is moved from $x_2$ to $y_4$, $d_3(x,y)=0.2$. $d(x,y)=d_1+d_2+d_3=0.5.
Im trying to define $d(x,y)$ very general. My first guess was to somehow combine the euclidean distance $\sqrt{(x_i-y_j)^2}$ and the euclidean distance $\sqrt{(i-j)^2}$ but it didnt worked out to well :) I would appreciate any help!