Bound on mutually x-ray-visible lattice points?

Question

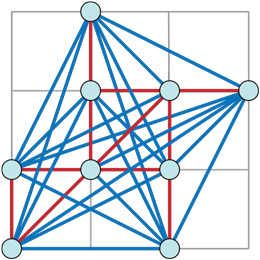
Say that two lattice points $a$ and $b$ of $\mathbb{Z}^d$ are $x$-visible to one another if the segment $ab$ contains at most $x$ lattice points (excluding $a$ and $b$). So $x$-visiblity is "x-ray visibility," with the power to see through $\le x$ blocking lattice points. For normal lattice visibility, $0$-visibility, there are at most $2^d$ mutually $0$-visible points, as established in an earlier question.

Q. For a given $x$ and $d$, what is the largest number of $\mathbb{Z}^d$ lattice points that are mutually $x$-visible?

Call this number $g(x,d)$. For example, although $g(0,2)=4$, $g(1,2) \ge 9$ (now corrected):

---

         

---

Answer 1

The largest number is $g(x,d) = (x+2)^d$, by an analogous argument as for visibility: if the differences of all coordinates of two points $A,B$ are divisible by $x+2$, then there are at least $x$ lattice points in the interior of the segment $AB$.

The set $\{0,1,\dots, x+1\}^d$ gives the lower bound.