Let $h(n)$ be the largest number of mutually invisible points that can be located in a
polygon $P$ of $n$ vertices. Two points $x$ and $y$ are mutually invisible if the segment
$xy$ contains a point strictly exterior to the closed set $P$.
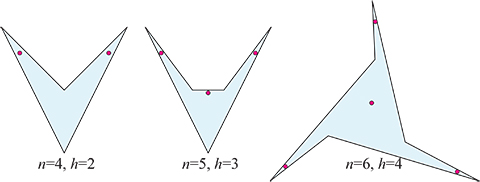
I have a vague sense that this question—What is $h(n)$?—may have been studied before, and perhaps even entirely resolved, but I am not recalling references. Any help would be appreciated—Thanks!
Answered by Wlodek Kuperberg:

The same construction shows that $h(n)=n-3$ for hiding points in a polyhedron of $n$ vertices in $\mathbb{R}^3$.
