Oscillator with discrete number of amplitudes?

Question

I know the question is a little vague, but I would like to know if someone can direct me to what kind of oscillator (if exist), that can follow the next behavior.

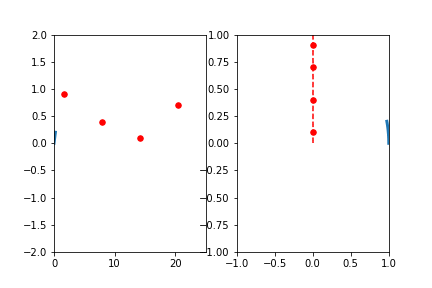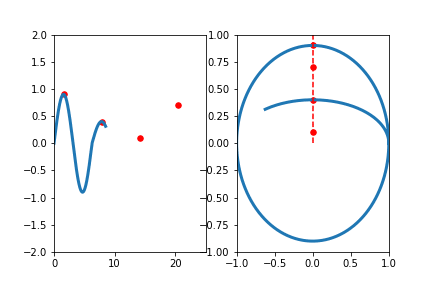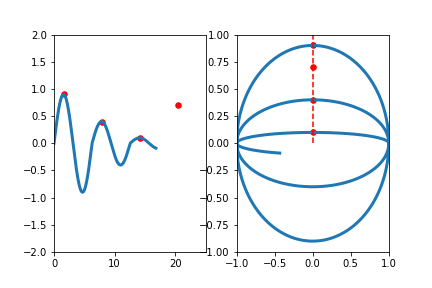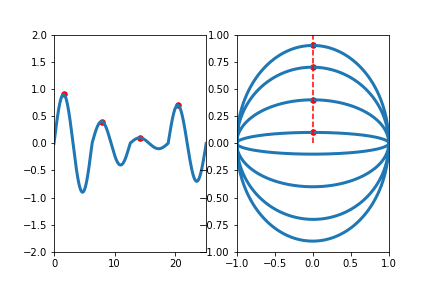
I manually create the gif to try to explain the problem.

It's a wave with a discrete number of maximum amplitudes. In the example there are four of those points on the red line.

What I have in mind is probably a chaotic oscillator that always cuts a line or plane (in the graph the Y axis) on the same points.

I have checked information about chaotic oscillators, and also some information about quasiperiodic motion, but I need some help on how to proceed or what to keep looking.

In the end, what I really want is a chaotic oscillator that cuts a line or plane, in only some discrete number of points. Is it possible?

Hope it makes sense.

Thanks!

Answer 1

I'm not sure about what your requirements are. In particular, I didn't get whether you need that the phenomenon arises from a chaotic oscillator, or it was just your try to search among those.

However, if I understood well your requirements, the phenomenon is quite mundane in the solutions of nonlinear PDEs exhibiting soliton character. For instance, if you take the standard sine-Gordon equation $$\ddot{u}-u_{xx}+\sin u=0,$$ then you have the so called breather solutions which look like what you're searching for. You may look at the classic paper Ablowitz, Kaup, Newell, and Segur - Method for solving the sine–Gordon equation from Physical Review Letters.

Answer 2

I would say that what you are looking for is commonly referred to as mixed-mode oscillations (MMO) . For more, I would recommend looking at chapter 13 of the book "Multiple Time Scale Dynamics" by Kuehn. I would also agree with the comment by Alessandro Della Corte that you do not need the orbit to be chaotic to obtain this phenomena. Furthermore, if the orbit you have is in fact chaotic, then I believe that it cannot attain its local maxima at finitely many discrete points.