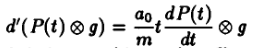Twisted affine Lie algebras, Lie bracket and normalized standard invariant form

Question

I am reading the book: Infinite-Dimensional Lie Algebras (Kac, third edition) and the article: Affine Lie algebras and the Virasoro algebras I (Wakimoto, link). The formulas they wrote for the Lie bracket $[,]$, normalized standard invariant form $(|)$ of twisted affine Lie algebras of type $X_N^{(r)}$ are contradicted to each other:

Contradiction1: In the book, page 139, the bracket is given by

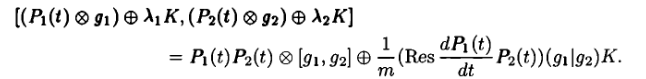
but in the article, page 381, it is given by

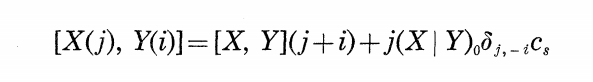
Here $X(j)$ means $t^j \otimes X$ and $c_s=rK/m$ (see the article to verify it). They are totally different.

Contradiction2: In the book, page 139, if the normalized standard invariant form is defined by

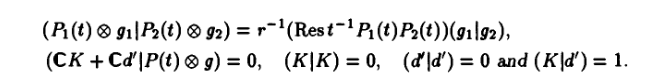
then it contradicts to the Lie bracket in the same page,

since $(d'| [t^i \otimes x, t^j \otimes y]) \ne ([d',t^i \otimes x]| t^j \otimes y)$

So, If are there anyone knows the right formulas for the Lie bracket and normalized standard invariant form for twisted affine Lie algebras mentioned in the Theorem 8.7 in the book of Kac?

Answer 1

I think that there is just a little mess between things that are denoted $K$, $K'$ in the book, as well as $d$, $d'$. For that, let us examine these formulas carefully.

Using the first formula for the Lie bracket, we get $$[X(j),Y(i)]=[t^j\otimes X, t^i\otimes Y]=t^{i+j}\otimes [X,Y]+ \frac{1}{m}\mathrm{Res}\frac{d x^j}{dt} x^i (X\mid Y) K,$$ which is equal to $$t^{i+j}\otimes [X,Y] + \frac{1}{m}\mathrm{Res} jx^{i+j-1} (X\mid Y) K=t^{i+j}\otimes [X,Y] + \frac{1}{m}j \delta_{i+j-1,-1} (X\mid Y) K,$$ and the latter is obviously equal to
$$ t^{i+j}\otimes [X,Y]+ \frac{j}{m}\delta_{j,-i} (X\mid Y) K, $$ while the second formula says $$ [X(j),Y(i)]=[X,Y](i+j)+j(X\mid Y)\delta_{j,-i}c_s=[X,Y](i+j)+j(X\mid Y)\delta_{j,-i}\frac{rK}{m}, $$ obviously equal to $$ t^{i+j}\otimes [X,Y]\oplus \frac{rj}{m} \delta_{j,-i} (X\mid Y) K, $$ which is obtained from the "totally different" formula by replacing $K$ with $rK$.

Now let us examine the second formula. We have $$ (d'\mid [P_1(t)\otimes g_1, P_2(t)\otimes g_2])=(d'\mid P_1(t)P_2(t)\otimes [g_1,g_2]+\frac{1}{m}\mathrm{Res}\frac{dP_1(t)}{dt}P_2(t)(g_1\mid g_2)K) $$ which is clearly equal to $$ \frac{1}{m}\mathrm{Res}\frac{dP_1(t)}{dt}P_2(t) (g_1\mid g_2), $$ since $d'$ pairs nontrivially only with $K$. At the same time, we have $$ ([d',P_1(t)\otimes g_1] \mid P_2(t)\otimes g_2)= (\frac{a_0}{m}t\frac{dP_1(t)}{dt}\otimes g_1\mid P_2(t)\otimes g_2), $$ which is equal to $$ \frac{a_0}{m}r^{-1}\mathrm{Res} t^{-1}t\frac{dP_1(t)}{dt}P_2(t) (g_1\mid g_2)= \frac{a_0}{m}r^{-1}\mathrm{Res}\frac{dP_1(t)}{dt}P_2(t)(g_1\mid g_2), $$ so it differs from the first formula by the scalar $a_0r^{-1}$.

I propose to you to look at page 131 of the book, which says, at the bottom, "It is also easy to see that $K=rK'$ is the canonical central element, and that $d=a_0r^{-1}d'$ is the scaling element". If you compare this sentence with my calculation above, you will be able to figure out what is going on, I am sure.