Akbulut's cork is the Mazur manifold $W$ shown in the picture below,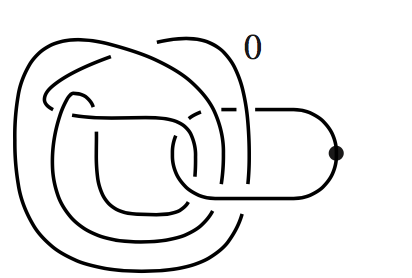
This manifold carries an involution of it's boundary $f:\partial W\to \partial W$ that exchanges a meridian of the 0-framed curve with a meridian of the dotted curve.
In Akbulut - 4-manifolds, the author says that $f$ is related to performing surgeries to exchange the dot with the 0-framed knot. I don't understand how this relates to $f$ and how $f$ is defined.
We can surely perform two surgeries (one along a circle and one along a 2-sphere) to get a new 4-manifold $\tilde{W}$ that has a Kirby diagram obtained by exchanging dot and zero in the above picture. However surgery does not affect the boundary so I do not see how this could induce a nontrivial map between the boundaries.
Precisely how is $f$ defined?
