Mednykh's formula for 2d manifold with boundaries? How to count principal G-bundles with prescribed holonomies?

Question

Let S be a closed, orientable 2d manifold and G a finite group. Since a principal G-bundle over S is specified by maps $\phi : \pi_1(S) \rightarrow G$ modulo the adjoint action by G, the way to count how many such bundles exist is \begin{align} \dfrac{ | Hom(\pi_1(S),G) |}{|G|} = \sum_{\text{irreps V of }G} \bigg( \dfrac{d(V)}{|G|} \bigg)^{2-2g} \; , \end{align} where $d(V)$ is the dimension of the irrep V, and g is the genus of S. My question is how the formula above generalizes when we want to count principal G-bundles over S, when S has n boundaries with prescribed holonomies for the bundle specified by conjugacy classes $\{ k_i : i=1,...,n \}$ of G. Morally we should have something like \begin{align} \dfrac{ | Hom(\pi_1(S_{g,k_i}),G) |}{|G|} = \sum_{\text{irreps V of }G} \bigg( \dfrac{d(V)}{|G|} \bigg)^{2-2g-n} \prod_{i=1}^n \chi_V(k_i) \; , \end{align} where $\chi_V(k_i)$ is the character of the representation V evaluated at any representative of the conjugacy class $k_i$. So, is the above formula completely correct? Are there factors missing? I would really appreciate any references that prove what the generalization is.

Answer 1

I get a slightly different formula:

$$\sum_{V} \frac{d(V)^{2-2g-n}}{|G|^{2-2g}} \prod_{i=1}^n |k_i|\chi_V(k_i)$$

Here $|k_i|$ denotes the size of the conjugacy class.

I prefer to express this in a slightly different way. For a conjugacy class $k$ and irreducible representation $V$ of $G$, let $f^V_k$ denote the scalar by which the indicator function $k \in Z(\mathbb C[G])$ acts on $V$. In these terms the formula is:

$$\sum_V \left(\frac{d(V)}{|G|}\right)^{2-2g} \prod_{i=1}^n f^V_{k_i}$$

To obtain the first formula from the second, note that the numbers $f^V_k$ are related to characters as follows:

$$f^V_k = \frac{|k|}{d(V)} \chi_V(k)$$

---

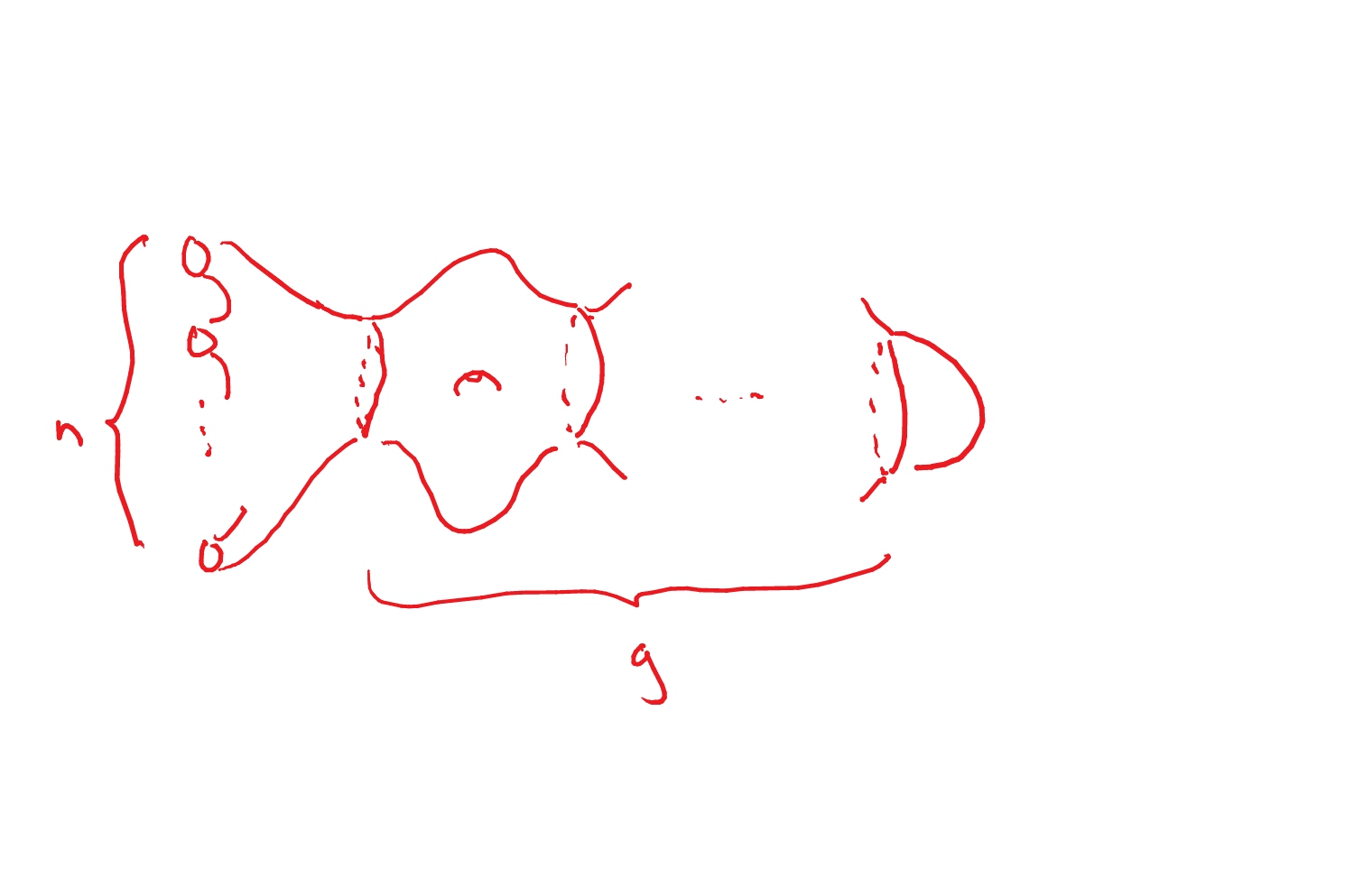
For me, these formulas come from general properties of commutative Frobenius algebras, or equivalently 2d topological field theory (TFT). Namely, given a commutative Frobenius algebra $A$, a genus $g$, and a collection of elements $k_1, \ldots , k_n$ one obtains a number

$$Z_A(g; k_1, \ldots , k_n)$$

recording the value of the TFT on an oriented surface of genus $g$ with $n$ punctures labelled by the elements $k_1, \ldots , k_n$. You can compute this number by first multiplying together the elements $k_1 \ldots k_n$ in $A$, then applying a sequence of $g$ comultipication followed by multiplication operations, finally followed by the Frobenius trace (see cartoon below).

In the case when $A$ is semisimple, one can be more explicit and write everything in terms of a basis of orthogonal idempotents.

In our case we take $A=Z(\mathbb C[G])$, the center of the group algebra, equipped with the trace $t$ (which takes the value $1/|G|$ at the identity element of $G$ and zero on all other elements). The numbers $f^V_k$ is just the change of basis matrix between the conjugacy classes $k$ and the orthogonal idempotents $e_V$ labelled by irreps.

I can't think of a reference for this right now. In this paper I explain some of this stuff in a related context, see e.g. Prop 2.13.