Embedded ribbons and regular isotopy

Question

I'm reading Kauffman's 1990 paper "An Invariant of Regular Isotopy" about knots that are isotopic through only Reidemeister Type II and III moves, which is known as a regular isotopy. His paper claims there is a relationship between regular isotopy and embedded bands ($S^1 \times [0,1]$) in $S^3$. He refers to Burde's Knots textbook, but I can't find any mention of regular isotopy, because it seems Kauffman coined that phrase in the paper, after Burde's text was written.

I think a regular isotopy of knots corresponds to an embedded band. However, I'm worried there might be a pathology I'm overlooking. Does anyone have a precise statement of the relationship?

Answer 1

From any knot diagram, one can obtain a framed knot by taking the "blackboard framing." The point of regular isotopy of knot diagrams is that it preserves this blackboard framing. Since framed knots and embedded bands are the same thing, regular isotopy will also preserve the embedded band corresponding to the blackboard framing of the knot diagram.

I assume this is discussed in more detail in Burde, maybe in terms of framed knots. It's also possible Burde doesn't discuss framed knots at all, since I think people became much more interested them after the discovery of the Jones polynomial/the Chern-Simons TQFT. And I agree: I think Kauffman coined the term "regular isotopy," so it is probably not used in Burde.

Answer 2

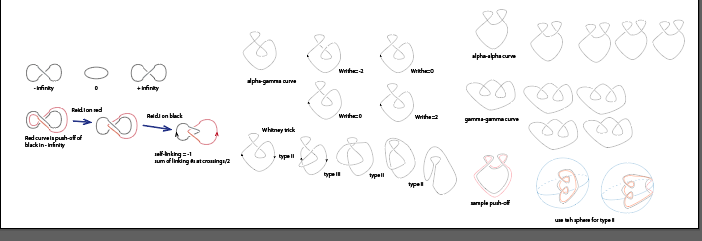
This is more of a comment than an answer, but I hope it's helpful. There is a much older and better-studied notion of regular homotopy. Let $X$ and $Y$ be smooth manifolds and let $f,g\colon X \rightarrow Y$ be immersions. Then $f$ and $g$ are regularly homotopic if they are homotopic through immersions.

Let's focus on regular homotopy classes of immersions $S^1 \rightarrow \mathbb{R}^2$. Such an immersion is what you get from a knot diagram by forgetting the over/under crossings. It is not hard to see that if $f,g\colon S^1 \rightarrow \mathbb{R}^2$ are regularly homotopic immersions with transverse self-intersections, then $f$ can be transformed into $g$ by a sequence of the obvious analogues of Reidemeister II/III moves. However, you can't perform an analogue of a Reidemeister I move since at the moment you pull your loop tight the derivative has to vanish, so it is not a regular homotopy.

My guess is that this is what Kauffman was thinking about. By the way, regular homotopy classes of immersions $S^1 \rightarrow \mathbb{R}^2$ can be completely classified. Taking the derivative of such an immersion and rescaling to make the derivative have unit length, you get an associated map $S^1 \rightarrow S^1$. The degree of this map is called the degree of the immersion, and the Whitney-Graustein theorem says that this degree is a complete invariant. This theorem is an early precursor to the Hirsch-Smale immersion theorem, which for the special case of immersions $S^2 \rightarrow \mathbb{R}^3$ includes Smale's famous "sphere eversions" that turns the sphere inside-out.

Answer 3

A diagram is drawn in the plane. Restrict to knots (not links). Orient the curve, & associate to each crossing a (+/-) via a Right hand rule: palm along over-crossing with pinky pointing towards orientation curl to +under-crossing. Thumb up= +sign. Sum over all crossings. This is the writhe. Writhe determines the self-linking of the knot with a push-off. Draw \infty+,\infty-, and 0. The \infty+ has the arc with +slope as the over-arc. Draw a push off curve in the plane, and compute the linking number <--tricky calc, best done by using RI moves to form Hopf link. The knot & a push-off bound an annulus. If the self-linking # of the knot is 0, then the annulus extends to a Seifert surface. The push-off defines a preferred longitude. But in general, the black-board framed curve has self-linking = writhe. With an \alpha -\gamma curve you can draw this in 4 ways. 2 have 0 writhe, 1 has +2, the other -2. The ones with 0 writhe are regularly homotopic to unknots. The other 2 require type I moves. Somewhere in Kauffman you'll see a Whitney trick.The alpha-gamma curve has 1 kink outward and 1 kink inward. There are alpha-alpha curves and gamma-gamma curves: two out or two in resp. In either case, the writhe can be arranged like a phone cord, or can cancel. The canceling cases are tricky. There the diags are on S^2.E.g. the bigon bounded in the gamma gamma case is on the outside. That's why you need to perform the framed isotopy in S^3 rather than R^3. [![0 and -/+ infinity curves