Let $T$ be a locally unknotted $2$-tangle in $B^3$ and $\Sigma(T)$ be its double branched cover. Can $H_1(\Sigma(T))$ have a non-trivial torsion? (Obviously, not for rational tangles.)
2 Answers
Studying this torsion has a nice application due to David Krebes (An obstruction to embedding 4-tangles in links, J. Knot Theory Ramif.,8 (1999), 321-352.) You can use it to show that a given 2-strand tangle doesn't embed as a subtangle of the unknot.
An example (similar to Ken's and related to Ian's comment): take the pretzel tangle P(-3,-3,3) drawn below; its double branched cover has homology $Z \oplus Z/3 \oplus Z/3$. This is from my paper (Embedding tangles in links, J. Knot Theory Ramif.,9, No. 4 (2000), 523-530).
Yes. For example, start with the exterior of the Whitehead link and do p/q filling on one component where p is odd. This manifold has p torsion and is the double branched cover of a two strand tangle in a ball.
You can see this by taking the quotient of the Whitehead link by the strong inversion and doing the appropriate rational tangle replacement on one component. The exterior of the other component (in the quotient) is then a two-strand tangle in the ball.
Note that if p were even, then you'd also have a single closed component in the tangle in addition to the two strands.
-
$\begingroup$ To clarify the construction: The point is that you're starting with a manifold that you know is the double branched cover of a 2-strand tangle (here, the solid torus) and doing p/q surgery on a strongly invertible null-homologous knot (here, a genus one knot in the solid torus). The surgery dual curve will represent torsion of order p. The quotient is a rational tangle replacement on a neighborhood of an arc with ends on the original tangle. Hence you'll get another 2-strand tangle, or one with an extra closed component depending on the parity of p. $\endgroup$ Commented May 4, 2020 at 13:33
-
$\begingroup$ I wouldn't be surprised if you already had a picture of this somewhere on your blog. $\endgroup$– Ian AgolCommented May 4, 2020 at 16:33

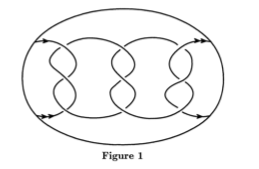 $(T_3)^* + (T_3)^* + (T_{-3})^*$">
$(T_3)^* + (T_3)^* + (T_{-3})^*$">