Denote the double branched cover of a $2$-tangle $T\subset B^3$ by $\Sigma(T)$. Since $\partial \Sigma(T)$ is a torus, I wonder if anyone studied the question: which knot complements in $S^3$ are of this form? We could call them "DBC knots". The unknot is one of them, however I suspect that that is a rather rare property and that for example torus knots are not DBC. But how one would prove it?
4 Answers
The complements of strongly invertible knots can always be realized as branched double covers of tangles. Torus knots are strongly invertible, so their complements actually do arise in this way.
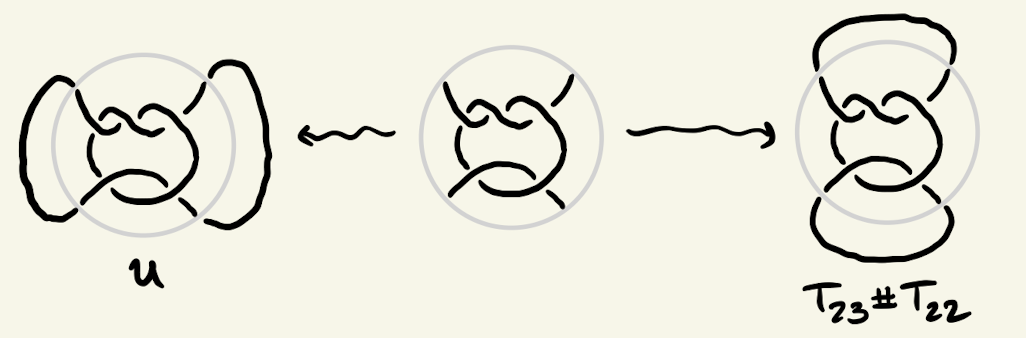
For torus knots there's an explicit construction which I learned from section 6 of Zentner's paper arXiv:1501.02504, though as he says it goes back to Montesinos. For example, here's a tangle $T$ to which we can glue one rational tangle to get an unknot, or a different rational tangle to get a connected sum of two-bridge links:
What this tells us is that the branched double cover $\Sigma(T)$ admits one Dehn filling to $S^3$, so it is the complement of a knot $K$; and that another Dehn filling of $\Sigma(T)$ is a connected sum of lens spaces, one of order $\det(T_{2,3})=3$ and one of order $\det(T_{2,2})=2$. In other words, $K$ has a $\pm L(3,1)\#\mathbb{RP}^3$ surgery.
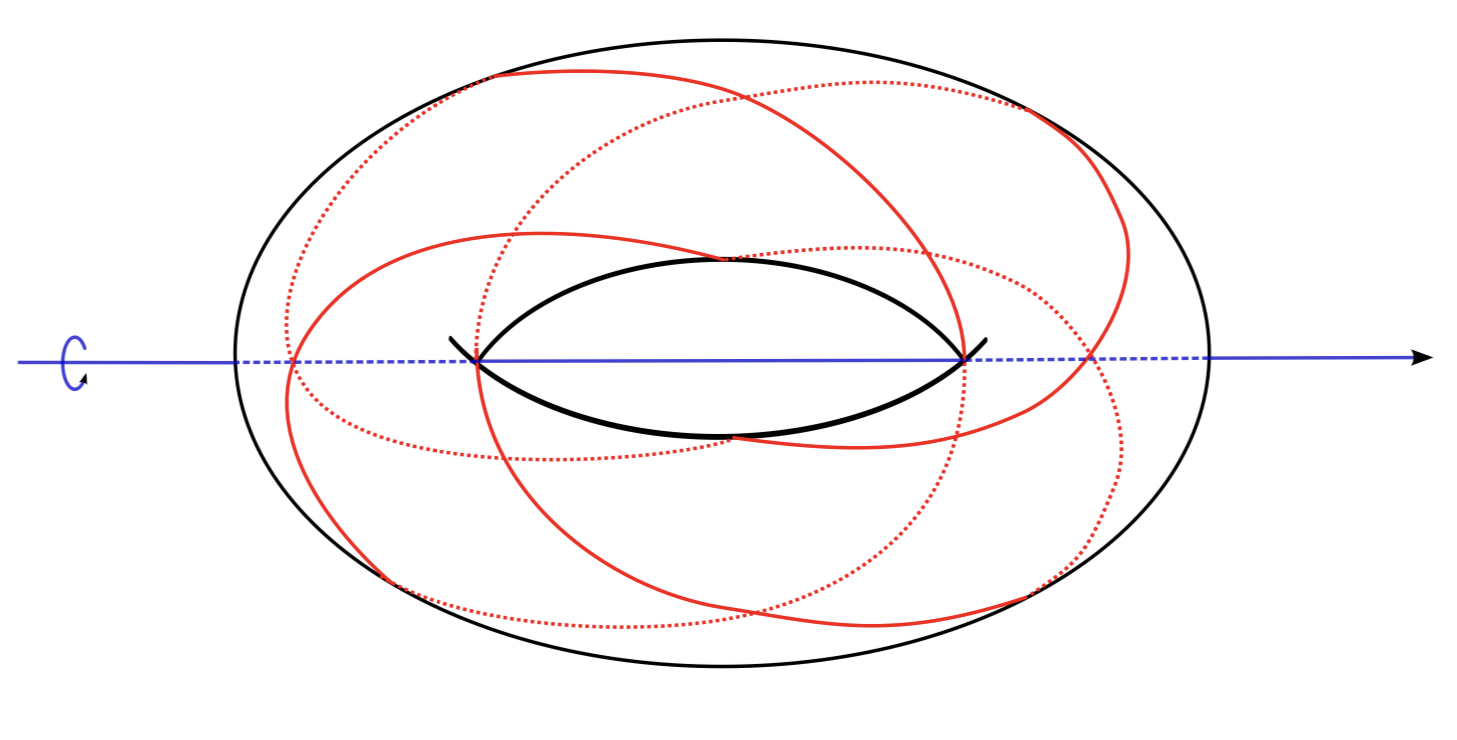
Now we know that $\Sigma(T)$ is Seifert fibered, so $K$ must be a torus knot, and then the reducible surgery above identifies $K$ as a trefoil. The reason $\Sigma(T)$ is Seifert fibered is that we got it from the branched double cover $S^1\times D^2$ of a trivial tangle by doing Dehn surgery on a pair of fibers of the product fibration. Here's a picture of that trivial tangle (in black) and some arcs (red and blue) which lift to fibers in the branched double cover; we got $T$ by replacing neighborhoods of the red and blue arcs with rational tangles, which amounts to Dehn surgery on those fibers in the branched double cover.

This procedure generalizes: you stick two rational tangles on top of each other to get $T$. Then $\Sigma(T)$ is Seifert fibered, with one Dehn filling being a connected sum of two lens spaces. If some other rational closure of $T$ is unknotted, then $\Sigma(T)$ must be the complement of a knot in $S^3$, and indeed of a torus knot since it's Seifert fibered. It's not obvious that you can find such tangles to get an arbitrary $T_{p,q}$, but Figure 4 of the linked paper above provides an explicit construction in terms of the continued fraction of $\frac{p}{q}$.
-
2
A couple of comments to supplement Steven Sivek’s answer.
There is a correspondence between strongly invertible knots with a given strong inversion and knotoids.
Your hypothesis of a double-branched cover of a tangle giving a knot complement implies that the knot complement admits an involution with fixed point set consisting of two arcs. On the boundary of the knot complement (peripheral torus), the involution acts as an elliptic involution, and hence extends over any Dehn filling, including the one giving back $S^3$. Hence the involution induces an involution of $S^3$. The Smith conjecture (a theorem) implies that the fixed point set of an orientation-preserving involution of the 3-sphere is an unknot. Thus there is an unknotted loop meeting the knot and an involution of $S^3$ fixing that loop and preserving the knot, reversing the orientation of the knot.
As for characterizing strongly invertible knots, likely the best one can hope for is an algorithm to recognize them. For hyperbolic knots, there are algorithms to compute the symmetry group and thus determine if it admits a strong involution. As mentioned in Sivek’s answer, torus knots are strongly invertible.
For the general case, one may use the JSJ decomposition. A strong involution will preserve the JSJ decomposition, and hence lead to an involution of each piece. The involutions of the Seifert pieces are understood (as in the torus knot case), and the involutions of the hyperbolic pieces can be computed. These can be assembled to determine if there is a global involution.
Classical invariants such as the Alexander and Jones polynomials seem to be not very good at obstructing strongly invertible knots since they do not detect knot orientation. For example, Sakai showed that any Alexander polynomial may be realized by that of a strongly invertible knot.
If a manifold $M$ is a double branched cover it admits a non-trivial involution and thus its mapping class group contains an element of order $2$. But in general, one expects a knot complement (or any other manifold) to not have any symmetries. In practice, this can for example be checked using SnapPy.
On the other hand, if one has a manifold $M$ that has an order $2$ element $f$ in its mapping class group (as for example some torus knot complements), then one can take the quotient $M/f$. If $M/f$ is $D^3$ then $M$ is a double branched cover over $D^3$. If $M/f$ is not $D^3$ for all involutions $f$ then $M$ is not a double branched cover over $D^3$ (but over a different manifold).
-
1$\begingroup$ What does it mean for a manifold to have an order 2 element in its complement? $\endgroup$ Commented Jul 25, 2022 at 8:14
-
1$\begingroup$ if the quotient is a ball then the involution reverses orientation, so the knot is amphichiral. Is the converse true? Or is there some other non-orientable degree-two orbifold quotient of $S^3$? $\endgroup$– HJRWCommented Jul 25, 2022 at 9:29
-
$\begingroup$ @MoisheKohan Yes, this was a typo. The order 2 element is in the mapping class group. $\endgroup$ Commented Jul 25, 2022 at 12:39
-
1$\begingroup$ In your second paragraph, an order 2 element of the mapping class group does not necessarily give an involution, so $M/f$ might be nonhausdorff if $f$ is not an involution (or finite order). $\endgroup$– Ian AgolCommented Jul 25, 2022 at 20:07
-
1$\begingroup$ @HJRW: for strongly invertible knots I imagine the involution $f$ as a 180-degree rotation about an axis which meets the knot twice. This is an oriented map $(S^3,K) \to (S^3,-K)$: it reverses the string orientation of $K$ but doesn't send it to the mirror $(-S^3,\pm K) \cong (S^3,\pm m(K))$, which is why $K$ need not be amphichiral. The quotient of the knot complement is obtained from $S^3/f \cong S^3$ by removing $N(K)/f \cong B^3$, so it's $B^3$. $\endgroup$ Commented Jul 25, 2022 at 20:56
There are several good answers above. I just wanted to provide an answer that addresses the concept of how 'rare' strongly invertible knots are. Of course, to say that these knots are 'rare' means that we have to assume some kind of random distribution of knots, set a threshold for what 'rare' means probabilistically (e.g. probability 0 as the complexity of the knots goes to $\infty$), and then show that this threshold is met somehow. It is tempting to perhaps look at knot tables and determine things that way, but all two-bridge knots and torus knots are strongly invertible and they tend to be over-represented among low-crossing examples.
To forgo defining and analyzing a random model for knots (largely because this leads to many non-canonical choices), I will try to outline some facts which exhibit the pressure points of having order 2 symmetries persist under surgery.
Let's consider knots obtained by surgery on a link of the form $L=K\cup C_1 \cup... \cup C_n$, where $K$ is a (possibly) knotted component and $C_i$ are all unknotted components such that $C_1\cup... \cup C_n$ is a split link. For example, $L$ could be a fully augmented link with one planar component. Then we can use a vector in $\mathbb{Z}^n$ (a_1,a_2,a_3...,a_n) to apply $1/a_i$ Dehn surgery along $C_i$ and obtain some knot in $S^3$.
We say the link $L$ is strongly invertible if there is an order 2 symmetry of $L$ which fixes points on every component of $L$. If $L$ is strongly invertible, then all surgeries will yield strongly invertible knots.
Let's now assume that $S^3 \setminus L$ is hyperbolic (and $L$ has the form as above). Consider what happens if $S^3 \setminus L$ admits an order 2 symmetry $\tau$ that exchanges at least two of the cusps. Say $C_i$ and $C_j$ are exchanged. In this case, for high parameter fillings along $C_i$ and $C_j$, fixing a filling of $C_i$ determines a unique filling on $C_j$ under the image of $\tau$. As a reference for this set of ideas,
Kojima, Sadayoshi, Isometry transformations of hyperbolic 3-manifolds, Topology Appl. 29, No. 3, 297-307 (1988). ZBL0654.57006.
and section 3 of my paper with Ken Baker and Joan Licata:
Baker, Kenneth L; Hoffman, Neil R; and Licata, Joan, Jointly primitive knots and surgeries between lens spaces, arXiv:1904.03268 [math.GT]
directly addresses when symmetries persist through this construction. Moreover, if there is a simple geodesic that is not fixed by a strong involution, then we can drill along that geodesic and its image to obtain an $S^3$ link complement with a symmetry $\tau$. As a word of caution, drilling might introduce components $C_{n+1}$ and $C_{n+2}$ that are knotted in $S^3$.