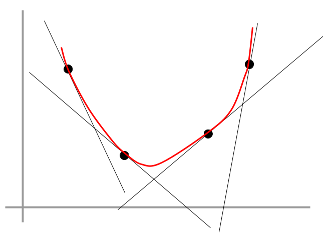
I guess my question is a follow up question of this one: usul, Existence of a strictly convex function interpolating given gradients and values, version: 2019-04-13.
In usul's question, the answer proves the existence of such a convex function. I think the assumption the question has - "For each point, we are given a linear function through it and strictly below the others" is very interesting. It's not difficult for us to construct such a convex function if the assumption is true. However, I am more interested in what conditions could make the assumption to be true?
In other words, I think my question is: suppose we are given a finite set $C$ with cardinality $T$ of pairs $(x,y) \in C$, with $x \in \mathbb{R}^d$ and $y \in \mathbb{R}$, under what conditions of $X = \{x_1, \dotsc, x_T\}$ and $Y = \{y_1, \dotsc, y_T\}$, can we construct $T$ linear functions $L =\{l_1, \dotsc, l_T\}$ such that each linear function $l_i$ passes through $(x_i, y_i)$ and is strictly below other points?
The mathematical formula for the above question may be written as the following: let each linear function $l_i = k_i(x - x_i) + y_i$, then the above question is to decide whether there exist a sequence of $K = \{k_1, \dotsc, k_T\}$ such that $l_i(x_i) > l_j(x_i)$, i.e., $$ y_i > k_j(x_i - x_j) + y_j \,\, \forall i\neq j. $$ Then I think the question is to decide the existence of such a sequence of $K$ which I am not sure about the answer. Also, I don't know if the above mathematical representation is the best way to solve this problem. Feel free to come up with your own method if my initial thinking doesn't work.
Updated: an image from usul's question so this question could be more intuitive: