As requested by Mathphile, since there have been efforts but no complete solutions to some questions raised when this question was asked on MSE, and since we think that here the question is more probable to be solved completely, I reproduce it here, in a different style.
The function $$r(b)=\sum_{k=1}^{\lfloor \frac{b-1}{2} \rfloor} (b \bmod{k})$$ is well-known from elementary number theory and has some well-known properties.
Mathphile considered the function $$z(m)=\sum_{n=1}^{m}(-1)^{r(n)}$$ and raised some questions, that still lack complete answer.
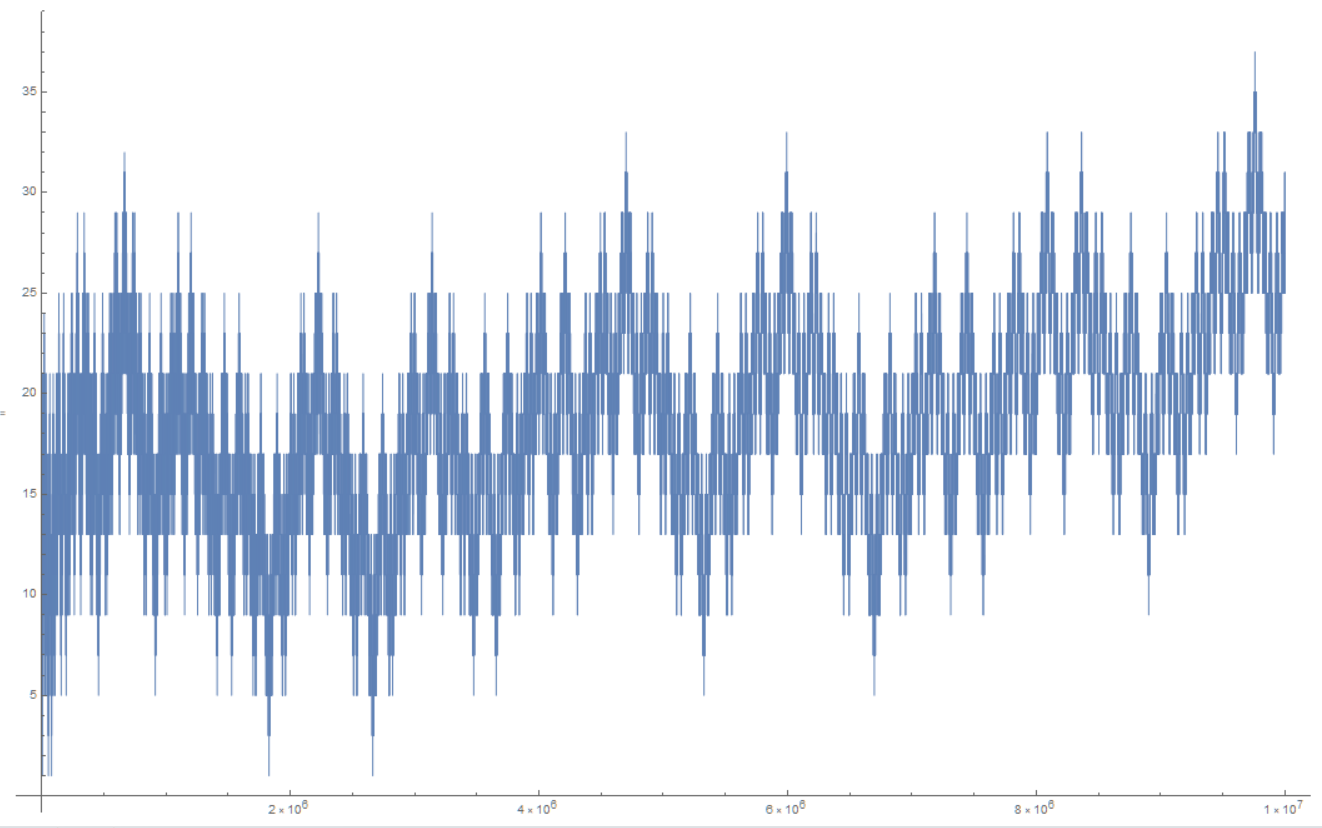
To get a feeling of how this function actually "behaves", here is the graph in some relatively large range, created by Vepir:
So, the function actually resembles almost-sinusoidal-fractal-like appearance and it seems to grow without bounds, although very very slowly.
The question is:
- Is $z$ unbounded?
Although this question has no direct application whatsoever, or at least I am not knowledgeable about it, it is nevertheless an interesting question awaiting for a solution, and we would like to see some complete solutions of this question.
Also, worth mentioning, is that although $z(m)$ returned back to $1$ a few times, interestingly enough we always had $z(m)>0$.
This was also question raised by Mathphile, namely, the question:
- Is $z(m)>0$ for every $m \in \mathbb N$?
So, these two questions are about what we would like to know about $z$.
Here is the question on MSE, along with some incomplete answers, but very skilled and detailed answers.
To evaluate the first few values, we took that $(-1)^0=1$.