Let $NS(T), D_+(T), D_-(T)$ denote closures of a $2$-tangle $T$ as in the picture. $T_0$ (below) is called trivial.
Question: Suppose that $NS(T)$ and $D_+(T)$ are trivial knots. Does it imply that $T$ is either $T_0$ or $T_2=$ the 2-braid with 2 negative crossings?
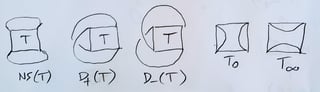
The branched double covers $\Sigma(NS(T))$ and $\Sigma(D_+(T))$ are both $S^3$ by assumption, and they're each built by gluing $\Sigma(T)$ to the branched double cover of a different rational tangle (i.e., a solid torus). This means that we can Dehn fill $\Sigma(T)$ along two different slopes to get $S^3$, so by the solution of the knot complement problem it must be an unknot complement. In other words, $\Sigma(T)$ is a solid torus, and so $T$ must be a rational tangle.
The closure $NS(T)$ is the "numerator closure" of $T$, and if its branched double cover is $S^3$ then $T$ must have fraction $\frac{1}{q}$ for some integer $q$, so $T$ is a 2-braid with some number $q$ of signed crossings. Now it's straightforward to identify $D_+(T)$ as the $(2,q+1)$ torus link, so this is only the unknot if q is 0 or -2, meaning if $T$ is either $T_0$ or $T_2$.
