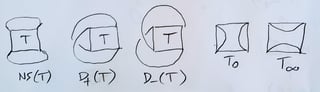
Let $NS(T), D_+(T), D_-(T)$ denote closures of a $2$-tangle $T$ as in the picture. $T_0$ (below) is called trivial. I conjecture
Question: Suppose that $NS(T)$ and $D_+(T)$ determine the triviality of $T$are trivial knots.
For Does it imply that consider framed tangles only, since otherwise $NS(T), D_+(T)$ are trivial both for$T$ is either $T_0$ and foror $T_2=$ the 2-braid with 2 negative crossings, and so $T_2$ can't be distinguished from $T_0.$
However, I conjecture that if $NS(T)$ is the framed unknot and $D_+(T)$ is the uknot with a positive kink, then $T$ is obtained from $T_0$, by adding framing $n$ to one strand and framing $-n$ to the other strand, for some $n$.
Would you have a suggestion for a proof?