-8
$\begingroup$

I am studying research paper " A note on odd zeta values " by Tanguy Rivoal and Wadim Zudilin .

Note-> This question has been closed 2 times on math.stackexchange . Earlier it was posted on MathOverflow but people here said it should be posted on math stackexchange. But they have closed it 2 times consecutively.

Please help I am badly struck on it.

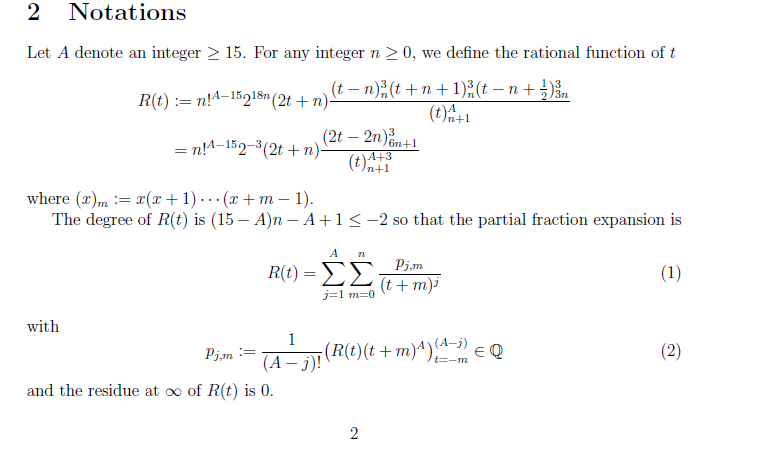
Notations: enter image description here

I couldn't think about how authors write the equation - we obtain $\phi_{(n) }^{-1} ( F(t) (t+m)^5 )_{ t=-m} $ $ \in \mathbb{Z} $ for m= 0 ,1,...,n by defininig $\phi_{(n)} = \prod_{2√n<p\leq n } p^{\rho_0(n/p) } $ .

Kindly see last 2 lines of image posted below , I have underlined(in black) the part in which i have question .enter image description here

enter image description here

I have understood everything in research paper till this argument but I don't have a clue on how authors derives the underlined part.

I think they are using [12, lemma1] (Paper-" One of odd zeta values from $\zeta(5)$ to $\zeta(25) $ is irrational by elementary means " By Wadim Zudilin )whose image I am posting below but unfortunately I am not able to obtain this relation from the lemma. Image of lemma ->

enter image description here

It would be really helpful for me if anyone can tell me how to deduce this equation $\phi_{(n) }^{-1} ( F(t) (t+m)^5 )_{ t=-m} $ $\in \mathbb{Z}$?

Improve this question
$\endgroup$
12
  • 3
    $\begingroup$ first of all, did you try to ask the authors? $\endgroup$
    – Fedor Petrov
    Commented Jan 25, 2020 at 8:23
  • 5
    $\begingroup$ Authors are almost always happy to answer questions from people interested in their work – the whole idea that someone is actually reading their paper is immensely satisfying. $\endgroup$
    – Gerry Myerson
    Commented Jan 25, 2020 at 8:55
  • 6
    $\begingroup$ Simulposted to m.se, math.stackexchange.com/questions/3521840/… without notice to either site. That's an abuse – please don't do that. $\endgroup$
    – Gerry Myerson
    Commented Jan 25, 2020 at 9:01
  • 4
    $\begingroup$ @Dxdxdade I downvoted because that's also a straightforward question -- just a matter of working out the notations. Please don't post on MO unless you have a serious doubt about an argument in a paper. Please consider using MSE instead. By the way, I don't like how you post the questions. It's better if you rewrite things in usual LaTeX without images. It will also benefit your understanding. $\endgroup$
    – François Brunault
    Commented Jan 25, 2020 at 11:21
  • 4
    $\begingroup$ @Dxdxdade By "serious doubt" I mean that you suspect that there is something wrong/incomplete in the argument, and you can substantiate that. So simply "not having any clue" is not enough to ask here. My advice would be that you rewrite the arguments (maybe taking particular cases and introducing your own notation) until you recognise which basic properties or theory you can apply. $\endgroup$
    – François Brunault
    Commented Jan 25, 2020 at 11:54

1 Answer 1

Reset to default
4
$\begingroup$

It's very easy (and this is probably why people are telling you that the question is not appropriate for MO).

You have a certain integer $X$, and you'd like to prove that $X$ is divisible by $\Phi_n$.

You know that $v_p(X)\geq\rho_0(n/p)$ for every odd prime $2\sqrt n\leq p\leq n$ (from the "Notations" section), meaning that $X$ is divisible by the $\rho_0(n/p)$-th power of $p$.

Hence you're done.

Improve this answer
$\endgroup$
1
  • $\begingroup$ here $\psi(t) $ = $\frac {\Gamma'(t) } { \Gamma(t) } $ and $\rho_0(t) $ is as defined in original question $\endgroup$
    – Arnold
    Commented Feb 11, 2020 at 17:38

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .