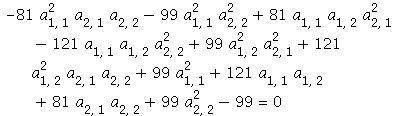Given the discrete-time Lyapunov equation (1):
$$ A^T P A - P = bb^T $$
such that $P$ shall be diagonal and positive definite and $b$ is a column vector. How to characterize $A$ and $b$, where there are diagonal solutions $P \succ 0$? More precisely,
$$ S = \{A \in \mathbb{R}^{n\times n}, b \in \mathbb{R}^{n} \mid \exists \textrm{ diagonal } P \succ 0 \textrm{ for } (1) \} $$
How to characterize $S$?
It seems hopeless to me. The considered equation
$A^TPA-P=bb^T$ can be rewritten $\phi(P)=bb^T$, where $\phi=(A^T\otimes A^T-I_{n^2})$ -if we stack the matrices row by row into vectors-.
If $spectrum(A)=(\lambda_i)_i$, then $spectrum(\phi)=(\lambda_i\lambda_j-1)_{i,j}$. Thus, in general , there is a unique solution
$P=(\phi)^{-1}(bb^T)$. On the other hand, since if $P$ is a solution, then $P^T$ too, $\phi^{-1}$ is an automorphism of the symmetric matrices.
Condition 1. The obtained symmetric $P$ is diagonal; there are $n(n-1)/2$ equations (dependent or not) linking the entries of $A=[a_{i,j}],b$. For example, when $n=2$ and $b=[88,-72]^T$, the condition is
When $n=3$, writing conditions takes up a lot of space!
Condition 2. $P>0$. That certainly works if the $(|\lambda_i|)_i$ are $>1$.
I make a different attempt to answer the question. $A,b \in S$ if there exists $c$ and $d$ such that
$$ \begin{bmatrix} A & b \\ c & d \end{bmatrix} \begin{bmatrix} P & 0 \\ 0 & 1 \end{bmatrix} \begin{bmatrix} A^T & c^T \\ b^T & d \end{bmatrix} = \begin{bmatrix} P & 0 \\ 0 & 1 \end{bmatrix} $$
This is equivalent to $$ \begin{bmatrix} P^{-1/2} & 0 \\ 0 & 1 \end{bmatrix} \begin{bmatrix} A & b \\ c & d \end{bmatrix} \begin{bmatrix} P^{1/2} & 0 \\ 0 & 1 \end{bmatrix} $$ being orthonormal. Thus $A$ is a submatrix of an (diagonally conjugated) orthonormal matrix. By Theorem 2.1 in Fiedler,1996 $A$ has at least $n-1$ singular values equal to 1 and the remaining one is less than 1. Diagonal conjugation may change this of course.
