1
$\begingroup$

As a follow up of the question raised in Determinant involving traceless unitary hermitian matrices, I would like to pose a similar question.

If A and B are distinct traceless unitary hermitian matrices, and S = A.B, the eigenvalues of S are always non-real?

EDIT: The counter-examples in the answers are relevant, but the matrices A and B in my problem satisfy other criteria. Simply being distinct is clearly not enough for have all eigenvalues of S as non-real. Therefore, let me add a second constraint:

To be more specific about the distinction between the A and B matrix, they cannot commute $[A,B] \neq 0$, and they cannot be block diagonal (irreducible).

This is surely true if A and B anticommute, {A,B}=0, which makes S skew-hermitian. However, this is a sufficient, but not necessary condition.

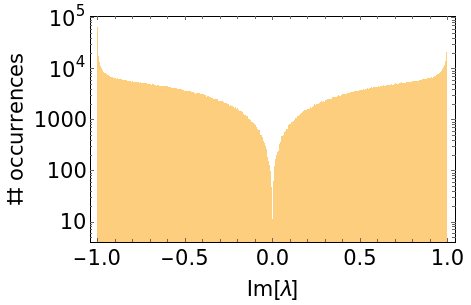
I've run a computer experiment on Mathematica to test this hypothesis, and it seems to hold, as shown in the figure in the link below. Within this numerical test, the smallest absolute value found for the imaginary part was 0.00046227, which is far from a numerical zero.

Histogram of the imaginary values of the eigenvalues of 1000000 sampled matrices S:

image

Improve this question
$\endgroup$
10
  • $\begingroup$ Have you tried algebraically testing the 2 by 2 case? $\endgroup$
    – user44191
    Commented Oct 18, 2019 at 17:42
  • 3
    $\begingroup$ No. Take $B=-A$, making $AB=-A^2$ symmetric. $\endgroup$
    – Christian Remling
    Commented Oct 18, 2019 at 18:27
  • 5
    $\begingroup$ If there is a trivial counterexample to your question, then it is the fault of the question, not the counterexample. You need to have a well defined question before you can expect people to invest effort in answering it. $\endgroup$
    – LSpice
    Commented Oct 18, 2019 at 19:46
  • 1
    $\begingroup$ @LSpice, I agree, of course. I'm working on the constraints for the A and B matrix that lead to S having all eigenvalues non-real. In the original question I've wrote that A and B should be distinct, but it is clear now that I should be more specific on this constraint. I'll edit the question to include that $[A,B] \neq 0$, and that they are irreducible (not block diagonal). $\endgroup$
    – Gerson J Ferreira
    Commented Oct 18, 2019 at 21:40
  • 2
    $\begingroup$ I’d just like to note that the numerical evidence here is not really indicative anything, since you’re essentially asking whether or not there exist matrices in some set of measure 0. It’s analogous to asking whether or not every matrix is invertible and then using the fact that every matrix you generate randomly is invertible as evidence of the affirmative. $\endgroup$
    – Nathaniel Johnston
    Commented Oct 18, 2019 at 22:26

1 Answer 1

Reset to default
4
$\begingroup$

Here is a counterexample:

$$A=\left( \begin{array}{cccccc} 0 & 1 & 0 & 0 & 0 & 0 \\ 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & -1 & 0 \\ 0 & 0 & 0 & 0 & 0 & -1 \\ \end{array} \right),\;\;B=\left( \begin{array}{cccccc} 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & -1 & 0 \\ 0 & 0 & 0 & 0 & 0 & -1 \\ \end{array} \right),$$ eigenvalues of $AB$ are $\{-1,-1,1,1,1,1\}$.

Addendum: The OP has added two more conditions on $A$ and $B$: they should not commute and they should not be block-diagonal; here is a counterexample that meets these new conditions as well:

$$A=\left( \begin{array}{cccc} 0 & 0 & 0 & -1 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & -1 & 0 \\ -1 & 0 & 0 & 0 \\ \end{array} \right),\;\;B=\left( \begin{array}{cccc} 0 & 0 & 0 & i \\ 0 & -1 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ -i & 0 & 0 & 0 \\ \end{array} \right),$$ eigenvalues of $AB$ are $\{-1,-1,i,-i\}$.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thanks! In this case [A,B] = 0, I'll have to add this constraint... A and B cannot commute. $\endgroup$
    – Gerson J Ferreira
    Commented Oct 18, 2019 at 19:47
  • 4
    $\begingroup$ This would not change the problem significantly. You can take Carlo's example as the first block of a block-diagonal example and add second diagonal blocks so that the overall matrices do not commute. The eigenvalues of the example here would still show up. $\endgroup$
    – Fabian Wirth
    Commented Oct 18, 2019 at 20:09
  • $\begingroup$ @FabianWirth, I agree. I've edited the question to improve the distinction between A and B. Let's consider then irreducible. In the block diagonal form is always possible to find a counter example. $\endgroup$
    – Gerson J Ferreira
    Commented Oct 18, 2019 at 21:48
  • $\begingroup$ @GersonJFerreira --- I have added a counterexample that meets the two criteria you added ($A$, $B$ noncommuting and not block diagonal). $\endgroup$
    – Carlo Beenakker
    Commented Oct 19, 2019 at 8:51
  • $\begingroup$ Thanks! Indeed my conditions for A and B is not sufficient. I could rephrase the question to ask what are the conditions on A and B (beyond those already stated) that constrains S to have only non-real eigenvalues? But this seems difficult to answer. We'll try to work around this issue. Thanks again! $\endgroup$
    – Gerson J Ferreira
    Commented Oct 20, 2019 at 18:12

Not the answer you're looking for? Browse other questions tagged .