14
$\begingroup$

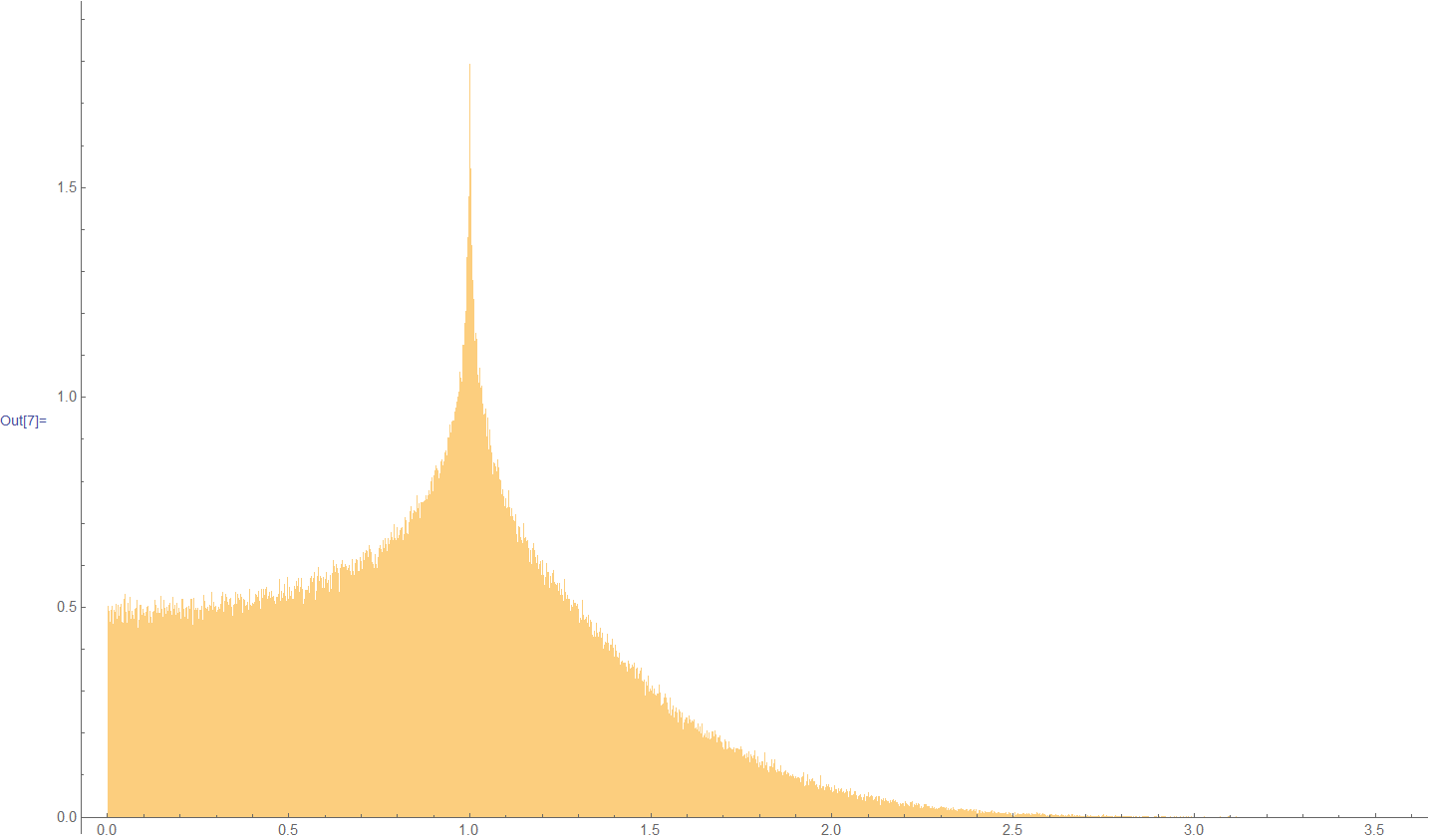
What is the large-n limit of a distribution of the following sample statistic:$$x\equiv\displaystyle\frac{\sum^{n}X_{i}}{\,\sqrt{\,\sum^{n}X_{i}^{2}\,}\,}$$ when sampling the Cauchy(0,1) distribution? Monte Carlo simulation indicates that convergence to this limit is quite fast, and that the resulting (symmetric) PDF has very sharp cusps at -1 and +1, but of course does not yield an analytic expression for this PDF - would anyone be able to find it?
Here is how the positive half of the PDF looks like:

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ A somewhat random remark: the limiting distribution is also the distribution of $X_1 / \langle X\rangle_1$ if $X_t$ is the Cauchy process and $\langle X\rangle_t$ is the quadratic variation process, a $\tfrac{1}{2}$-stable subordinator. I bet someone has studied the joint law of $X_t$ and $\langle X\rangle_t$, but unfortunately I do not have time now to search for the reference. $\endgroup$
    – Mateusz Kwaśnicki
    Commented Jun 16, 2019 at 20:17

1 Answer 1

Reset to default
16
$\begingroup$

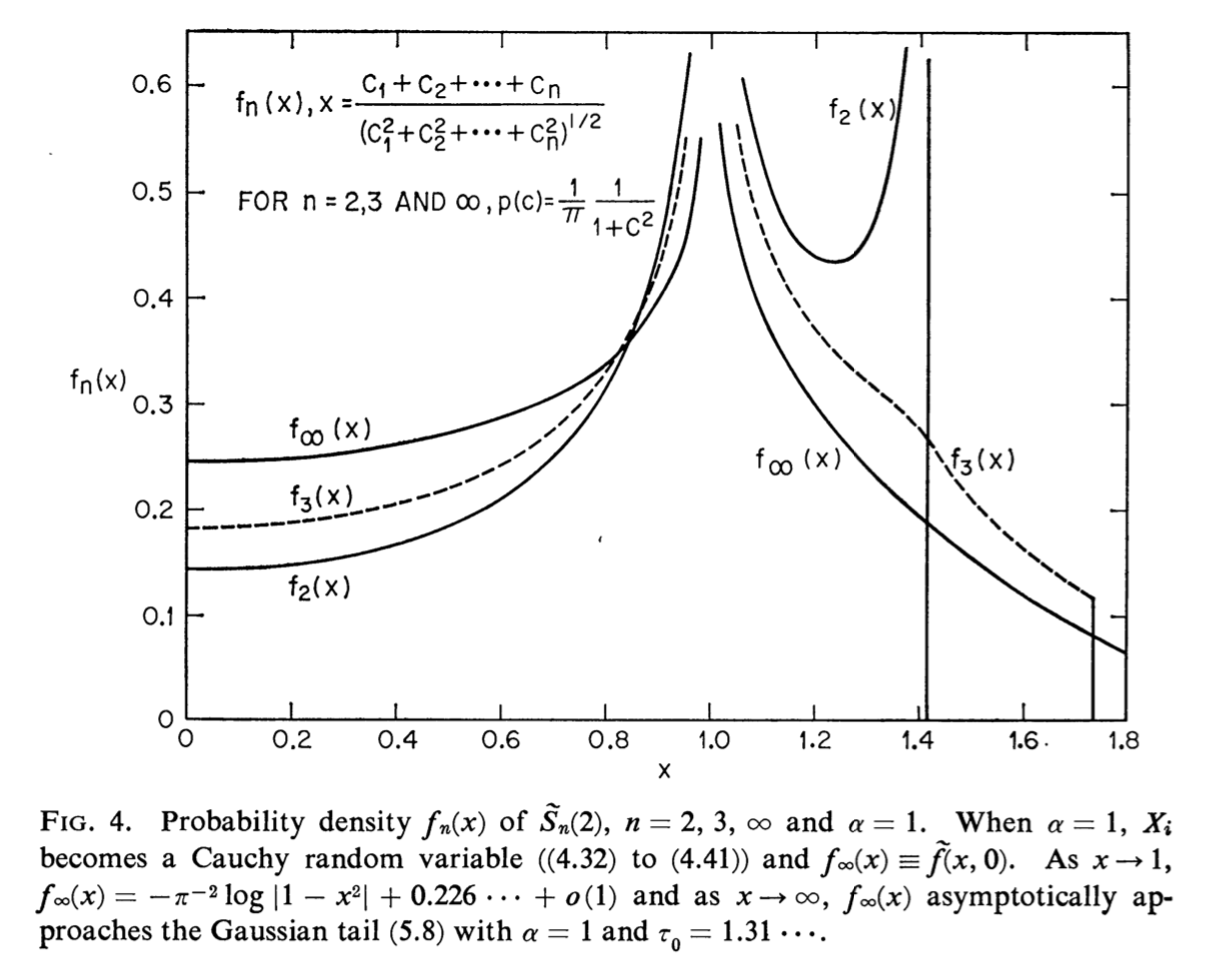
This desired large-$n$ limit of the distribution $P_n(x)$ is calculated in Limit Distributions of Self-normalized Sums (1973). The Cauchy distribution is the case $\alpha=1$ on page 798. The singularity in $\lim_{n\rightarrow\infty}P_n(x)$ at $x=\pm 1$ is logarithmic $$P_n(x)\rightarrow-\pi^{-2}\log|1-x^2|,$$ see equation (5.12) and figure 4 (reproduced below), while for large $|x|$ the decay is Gaussian.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for your help; the article you mention is as exhaustive as it could be - one has to accept that the asymptotic PDF is not a "pretty" function! $\endgroup$
    – Honza
    Commented Jun 16, 2019 at 22:29

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .