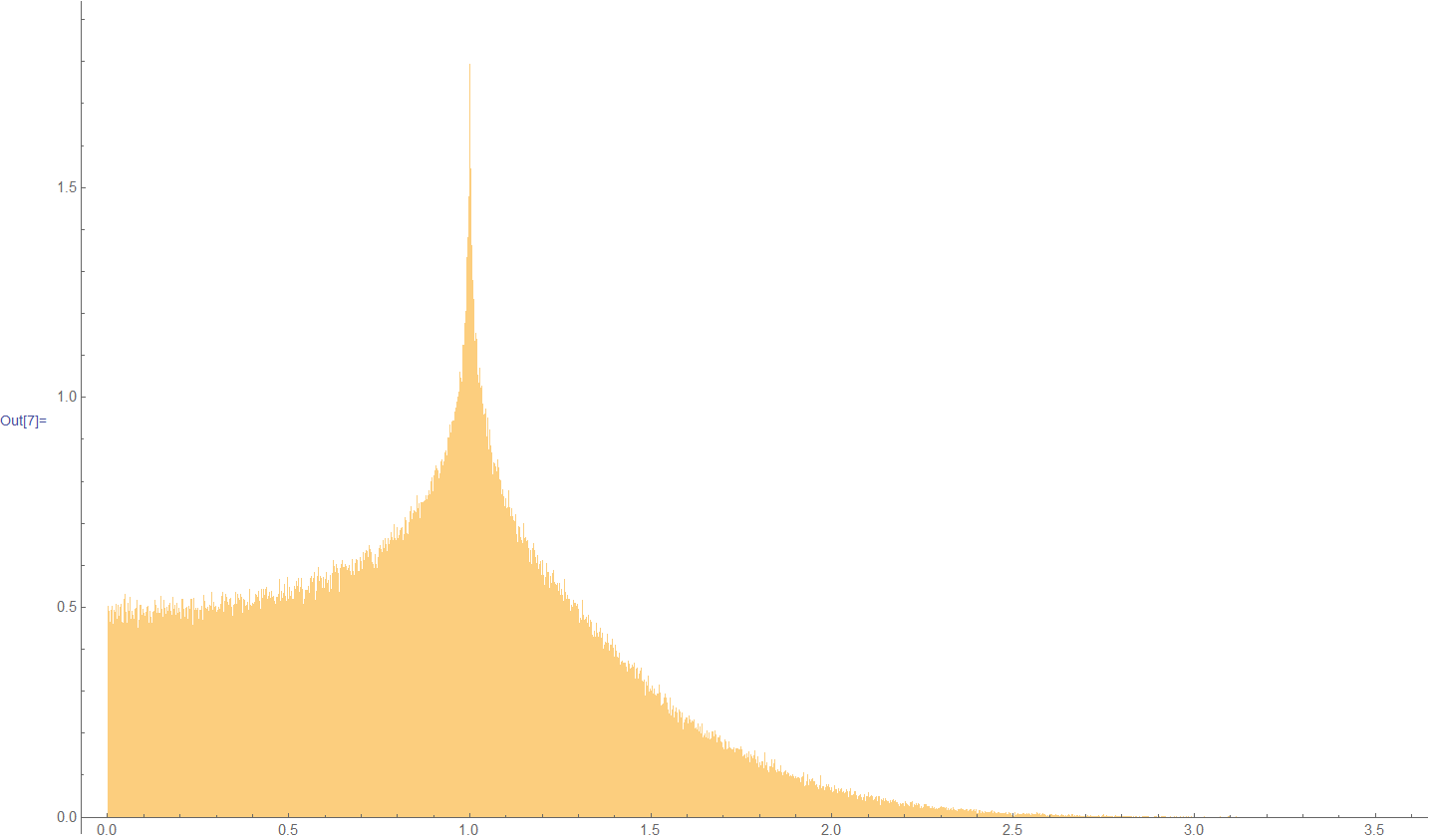
What is the large-n limit of a distribution of the following sample statistic:$$\displaystyle\frac{\sum^{n}X_{i}}{\,\sqrt{\,\sum^{n}X_{i}^{2}\,}\,}$$$$x\equiv\displaystyle\frac{\sum^{n}X_{i}}{\,\sqrt{\,\sum^{n}X_{i}^{2}\,}\,}$$ when sampling the Cauchy(0,1) distribution? Monte Carlo simulation indicates that convergence to this limit is quite fast, and that the resulting (symmetric) PDF has very sharp cusps at -1 and +1, but of course does not yield an analytic expression for this PDF - would anyone be able to find it?
Here is how the positive half of the PDF looks like:
Became Hot Network Question