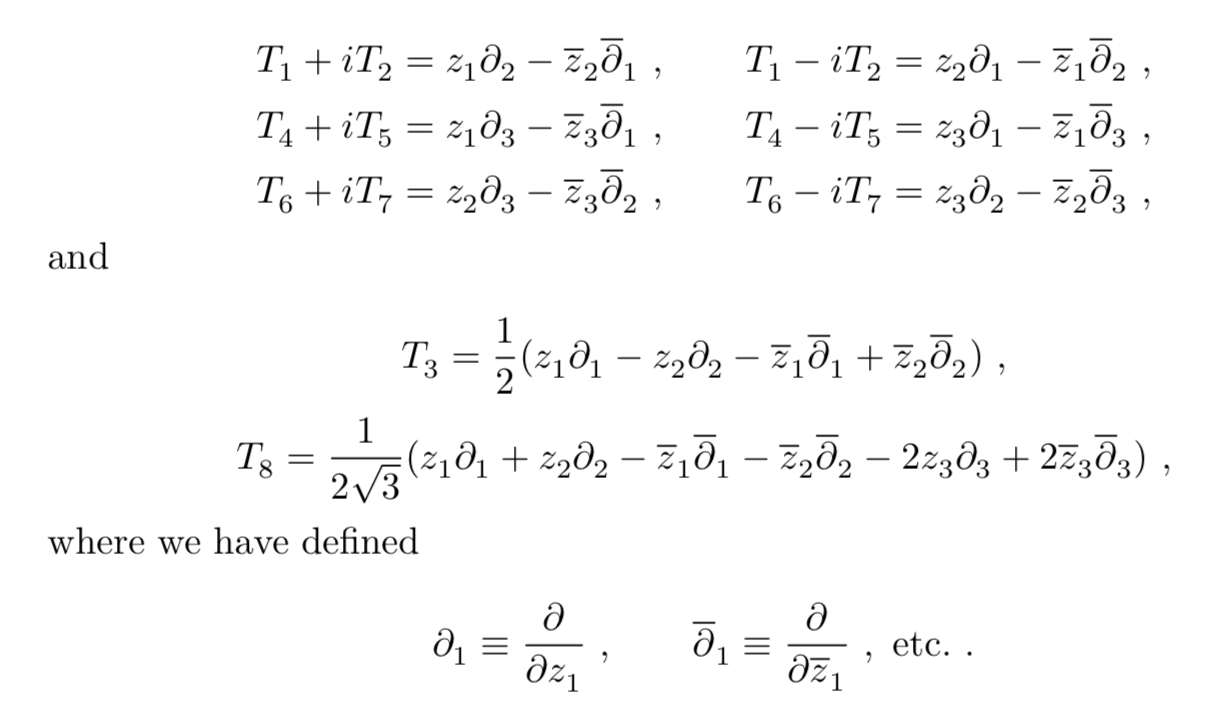Definition of a Dirac operator

Question

So it seems that a Dirac operator acting on spinors on $\psi=\psi(\mathfrak{su}(2),\mathbb{C}^2)$ can be written in this case simply as:

$D=\sum_{i,j} E_{ij}\otimes e_{ji}$, where $E_{ij}$ are elements of $U(\mathfrak{g})$ and $e_{ji}$ are elements of a Clifford algebra.

In the easiest form, $D=\sigma_x\partial_x+\sigma_y\partial_y+\sigma_z\partial_z$, where sigmas are the Pauli matrices for $su(2)$ on Euclidean space.

There's a cubic Dirac operator by Kostant, it reads something like $D=\sum_{i,j} E_{ij}\otimes e_{ji}+\sum_{ijkl}^{(2)}\otimes (e_{ij}\wedge e_{kl})$ but I am a bit unsure of what's $E_{ijkl}$ in this case, and how it is constructed. Could anyone write it "in the easiest form" as I did for $su(2)$?

For higher order, I'm guessing it is written in the exterior algebra of $\mathbb{C}^n$. Do you have any clue of matrices are these and how they are constructed?

Thank you!

Answer 1

An explicit representation of Kostant's equation over $SU(3)/SU(2)\times U(1)$ is given in Supersymmetry and Euler Multiplets. It is given in terms of Dirac matrices $$\{\gamma^a,\gamma^b\}=2\delta^{ab},\;\;a,b=4,5,6,7$$ and $SU(3)$ generators $T_n$, $n=1,2,\ldots 8$ by $$\sum_{a=4,5,6,7}\gamma^a T_a\Psi=0$$ where the generators have the following representation as differential operators:

The four resulting differential equations are