One can answer your question positively, although I haven't tried to compute $g_R$ as a function of $R$.
One can tessellate a certain right-angled pentagon by $238$ triangles.
Moreover, a genus 2 surface is an index 8 orbifold cover of the right-angle pentagon orbifold (i.e. an index 8 torsion free subgroup of the reflection group in a right-angled pentagon is a genus 2 surface group).

The area of a right-angled pentagon is $\pi/2$, and by Gauss-Bonnet the area of a genus $g$ surface is $4\pi(g-1)$, so one wants to find tessellations of a right-angled polygon by $g-1$ right-angled pentagons. The reflection group in this polygon will be an index $g-1$ subgroup of the right-angled pentagon reflection group. Then the induced index 8 subgroup will be a genus $g$ surface group.
Thus, we'd like to know for a given $g$, what is the right-angled polygon tessellated by right-angled pentagons which contains a disk of radius $R$? Eight copies of this polygon will give a fundamental domain for the surface group, and hence have the properties you desire.
As in Peter Scott's proof of the residual finiteness of surface groups, given a disk of radius $R$, we may take its convex hull with respect to the lines in the pentagonal tiling.
This will take some number of pentagons which is roughly exponential in $R$. As $R$ increases, we will get a sequence of linear growth $R_n$ with tilings containing $s_n$ pentagon tiles and containing a disk of radius $R_n$. In this diagram, the polygons are made of a convex union of pentagons containing each circle (note, the pentagon made of 238 triangles has a different shape since it's not regular, this is just for demonstration, but would still yield $R_n$ up to quasi-isometry):
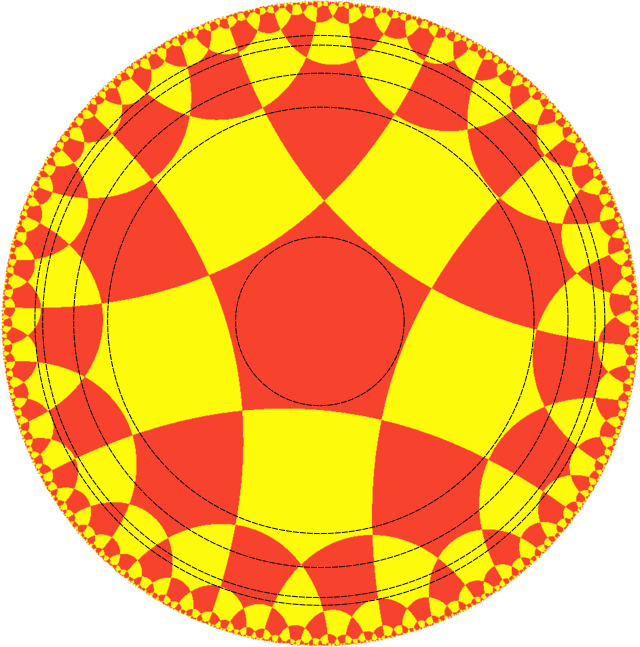
To interpolate between the $s_n$, a simple thing one can do is add a string of right-pentagons onto a free boundary component. Doing this, we can get any number of pentagons between $s_n$ and $s_{n+1}$, and hence for any number.


