Alternative characterization of epi-convergence

Question

I am struggling with the proof of a property of epi-convergence.

We need the following definitions:

For a sequence of sets $(C^\nu)_\nu$ in $\mathbb R^n$, the outer limit is the set $\limsup_\nu C^\nu = \{x:~ \exists N \in \mathcal N_\infty^\#, \exists x^\nu\in C^\nu:~ x^\nu\stackrel{N}\to x\} $. The inner limit is the set $\liminf_\nu C^\nu = \{x:~ \exists N \in \mathcal N_\infty, \exists x^\nu\in C^\nu:~ x^\nu\stackrel{N}\to x\} $

Here, $\mathcal N_\infty^\#$ is the set of subsequences of $\mathbb N$ and $\mathcal N_\infty$ is the set of "tails" of $\mathbb N$, i.e. sets of the form $\{M,M+1,M+2,\ldots\}$.

Also, the lower and upper epi-limit of a sequence of functions $f^\nu: \mathbb R^n\to \mathbb R$ is defined as (first by their epigraph):

$$ epi( e-\liminf_\nu f^\nu) := \limsup_\nu (epi (f^\nu))\qquad \text{ ( = outer limit of epigraphs)}$$ and

$$ epi( e-\limsup_\nu f^\nu) := \liminf_\nu (epi (f^\nu))\qquad \text{ ( = inner limit of epigraphs)}$$

Then we can define $e-\liminf_\nu f^\nu$ and $e-\limsup_\nu f^\nu$ by extracting the graph from the epigraph. If those two functions coincide, we call this the epilimit $e-\lim_\nu f^\nu$.

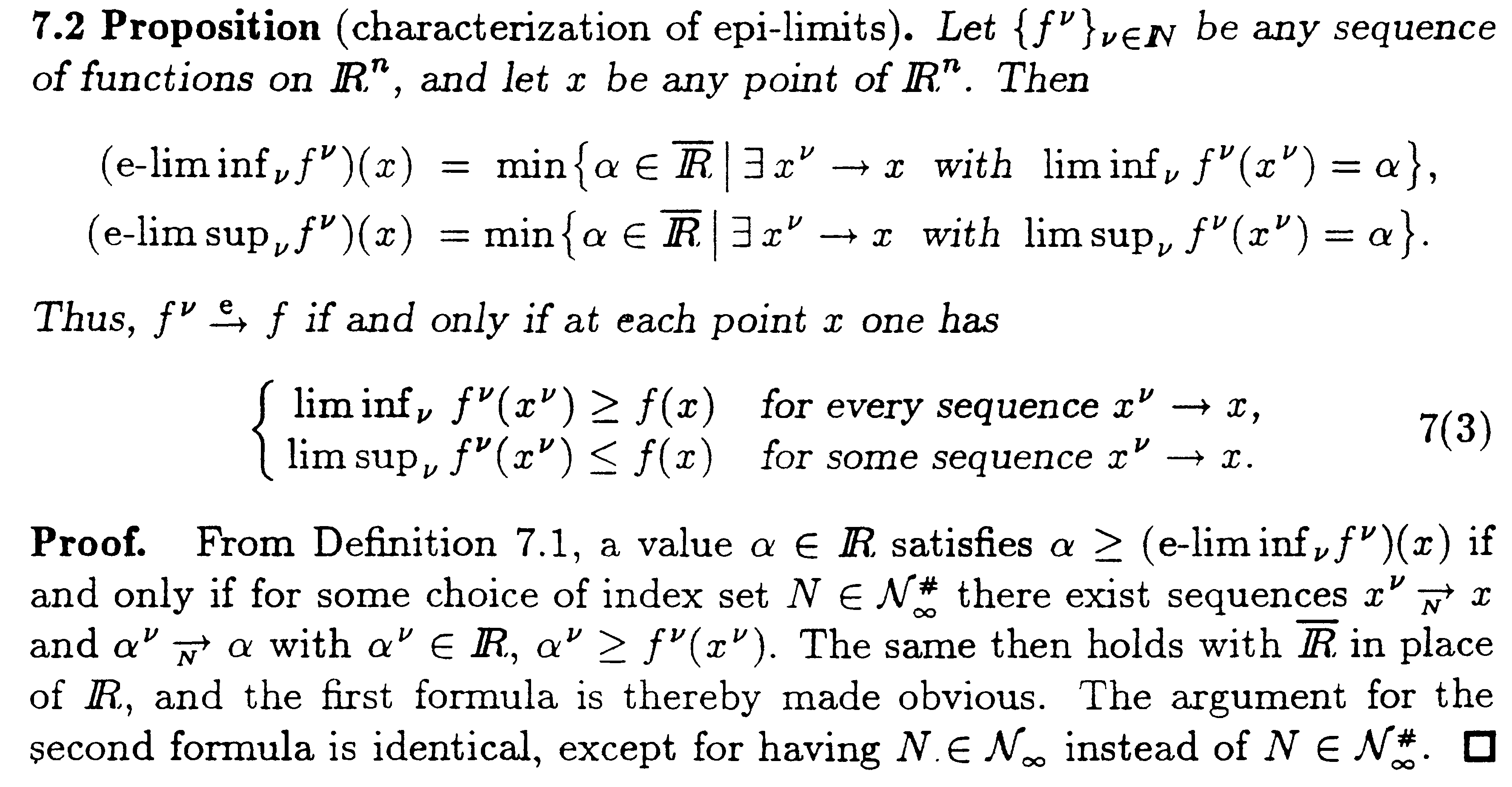
My problem is now the proof of the following proposition (from Rockafellar, Wets, "Variational Analysis", Chapter 7, page 241):

Now I don't understand at all everything from "the first formula is thereby obvious".

Even if we have $N\in \mathcal N_\infty^\#$ and $x^\nu \stackrel{N}{\to} x$, $\alpha^\nu \stackrel{N}{\to} \alpha$, how do we construct a sequence $x^\nu \to x$?

And even if the first two equations in the proposition are proven, how do we obtain equation 7(3) from there?

Answer 1

Partial Answer:

Even if we have $N\in \mathcal N_\infty^\#$ and $x^\nu \stackrel{N}{\to} x$, $\alpha^\nu \stackrel{N}{\to} \alpha$, how do we construct a sequence $x^\nu \to x$?

You can construct any sequence you want as long as it contains that subsequence and still converges to $x$. Because of the $\liminf$, this will suffice: If we have $\alpha^\nu\geq f^\nu(x^\nu)$ for a subsequence $N$, then it follows that $$ \alpha = \liminf_{\nu\in N} \alpha^\nu \geq \liminf_{\nu\in N} f^\nu(x^\nu) \geq \liminf_{\nu\in \mathbb{N}} f^\nu(x^\nu) $$ Here, the last inequality is true because we only add more elements to the sequence. This inequality implies that $\alpha$ is greater or equal to the right-hand side of the formula.

For the other direction, assume that $\alpha$ is greater or equal to the right-hand side of the formula. Thus, there is a sequence $x^\nu\to x$ with $\liminf_\nu f^\nu(x^\nu)\leq \alpha$. Now we choose the subsequence $N$ such that $f^\nu(x^\nu)\stackrel{N}{\to} \liminf_\nu f^\nu(x^\nu)$ and $\alpha^\nu := \max(f^\nu(x^\nu),\alpha)$. Thus, by the first "if and only if" statement in the proof, it follows that $\alpha\geq (e-\liminf_\nu f^\nu)(x)$.

Answer 2

Ok, so with help from supinf I managed to write a more complete version of the proof (which I can now understand). See https://pwacker.com/proof_epiconvergence.html for a hierarchical version of it.