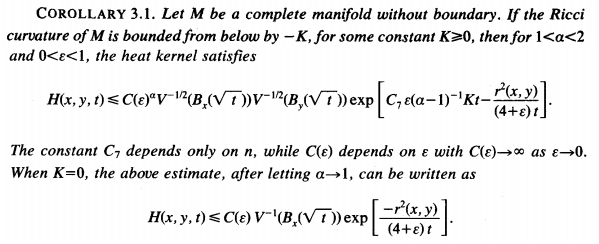5
$\begingroup$

Let $M$ be a compact Riemannian manifold without boundary, and let $\Delta$ be the Laplace-Beltrami operator on $M$. It is known that for small $t$, let's say, $0< t < t_0(M, g)$, the heat kernel $p(t, x, y)$ satisfies $$ p(t, x, y) \leq C_1 t^{-n/2} e^{\frac{-C_2d(x, y)^2}{t}}. $$

My question is the following. It seems intuitively that if one places certain curvature restrictions on $M$, the constants $C_1, C_2$ could be controlled. Is it known what kind of bounds we can have for $C_1, C_2$ (I am particularly interested in $C_1$) in terms of Ricci or sectional bounds? I looked up some literature, but there is very little I could find on compact manifolds. Any help would be appreciated. Thanks.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

I think that the closest statement to an answer is contained in the famous paper:

Li, Peter; Yau, Shing Tung, On the parabolic kernel of the Schrödinger operator, Acta Math. 156, 154-201 (1986). ZBL0611.58045.

In particular, in Corollary 3.1, they prove the following:

enter image description here

The estimate is global in time, and the constants are explicit in the proof. Furthermore, $V$ represents the volume of balls of radius $\sqrt{t}$. If you want to make them even more explicit in terms of $t$, you need to add an upper bound on the sectional curvature in order to bound from below the volume of balls with standard comparison theorems, at least for sufficiently small times.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Suppose we put some non-positive Ricci curvature bounds on $M$, by the Bishop-Gromov volume comparison theorem that should still imply that $C_1$ above should satisfy $C_1 \leq \frac{1}{(4\pi)^{n/2}} + \epsilon$, is that correct? At least this should work for small $t$, with $\epsilon \to 0 $ as $t \to 0$. $\endgroup$
    – user128124
    Commented Aug 24, 2018 at 17:34
  • $\begingroup$ Bishop-Gromov implies an upper bound on the volume of balls. You need the other way around. This is why I meantioned in my answer an upper bound on the sectional curvature, which is needed for a lower bound on the volume of balls. $\endgroup$
    – Raziel
    Commented Aug 25, 2018 at 9:06

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .