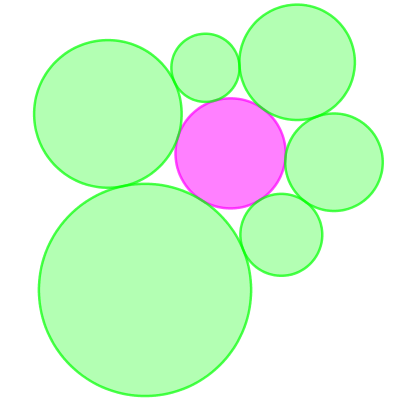
By my computation, I pose a conjecture as follows and I am looking for a proof:
Conjecture: Let $(O)$ be a circle with radius $R$, and $n$ be positive integer $n\ge 3$. Construct $n$ circles $(O_1), (O_2), \cdots, (O_n)$ external tangent to $(O)$ and $(O_i)$ tangent to $(O_{i+1})$ for $i=1$, $2$, $\cdots$, $n$ and $(O_{n+1}) \equiv (O_1)$. If radius of $(O_1)$, $(O_2)$, $\cdots$, $(O_n)$ are $r_1$, $r_2$, $\cdots$, $r_n$ respectively then
$$r_1+r_2+\cdots+r_n \ge \frac{n\sin^{\frac{\pi}{n}}}{1-\sin{\frac{\pi}{n}}} R$$
and
$$r_1^2+r_2^2+\cdots+r_n^2 \ge n\left( \frac{\sin{\frac{\pi}{n}}}{1-\sin{\frac{\pi}{n}}} \right)^2R^2$$
See also: