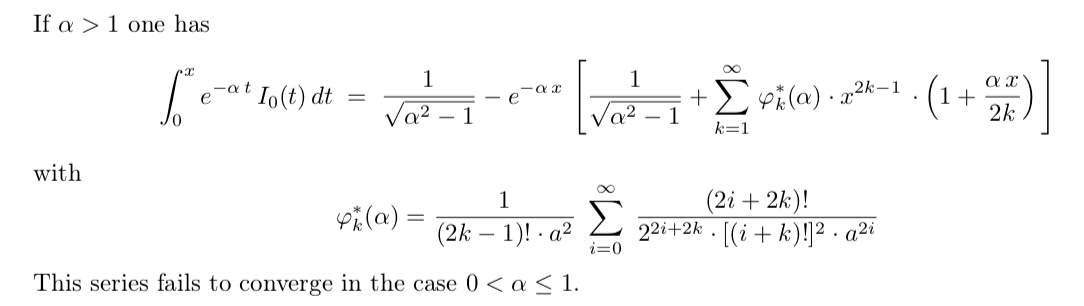Thanks to sound remarks here and here, and looking again at these equations, I noticed that my puzzle boils down to these 2 special functions: $$\int_0^x I_i(u) e^{-a u}du, \quad i=0,1$$ where $I_n(u)$ is the modified Bessel of the first kind.
Are these integral echoing something known in the literature? What can we know for $x<+\infty$ and $\alpha >1$? Especially for finite $x$, I know that it can be expanded in powers of $\frac{a^{-2}-1}{2}$ cf. here, probably hypergeometric series?
The integral is studied in On Certain Indefinite Integrals Involving Bessel Functions (1958).
(The $i=0$ integral is $g(a,0,x)$ in the notation of that paper, and as Robert Israel points out, the $i=1$ integral is simply related.)
The paper is behind a paywall, so I have not read it.
And then there is Tables of some indefinite integral of Bessel functions of integer order (2017), which examines the $i=0$ integral in great detail and gives several series expansions, see pages 87 and following.
I reproduce one such formula:
You might note that your two functions are closely related:
$$ \int_{0}^{x}\!{{ I}_{1}\left(u\right)}{{\rm e}^{-au}}\,{\rm d}u={ { I}_{0}\left(x\right)}{{\rm e}^{-ax}}-1+a\int_{0}^{x}\!{{ I}_{0 }\left(u\right)}{{\rm e}^{-au}}\,{\rm d}u $$
